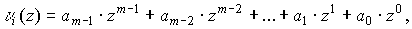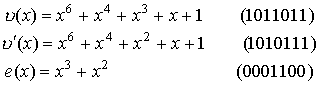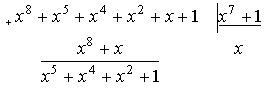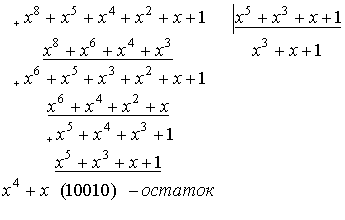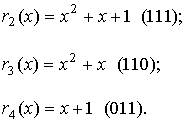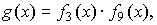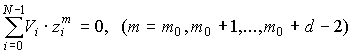В предыдущем параграфе было дано описание кодов Хэмминга как циклических кодов, порождающие многочлены которых имеют корень в соответствующем расширении основного поля. Описанные коды исправляют одну ошибку. Сейчас мы возвращаемся к исправляющим две ошибки кодам над полем GF (2). Пусть длина кода имеет вид 

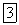
Пусть 


Принятое слово записывается многочленом степени 

где 












Пусть 








Если может произойти не более двух ошибок, то величина равна нулю тогда и только тогда, когда не произошло ни одной ошибки. Декодер должен продолжать работу в том случае, когда 

Прямого очевидного способа решения такой системы нет. Один из методов состоит во введении нового многочлена, определяемого так, чтобы локаторы ошибок были его корнями:

Если мы сумеем найти коэффициенты этого многочлена, то, разложив его на линейные множители, мы сможем найти 


Таким образом,

если произошли одна или две ошибки. Многочлен в правой части нам известен, поскольку известны 
Указав процедуру декодирования, мы установили, что выбранный многочлен 


Рассмотренные в настоящем параграфе коды, исправляющие две ошибки, служат иллюстрацией того, как построить
циклические коды над некоторым полем, используя лежащие в большем поле корни порождающих эти коды многочленов. В гл. 7 будет рассмотрен общий случай такого построения для произвольного поля символов 
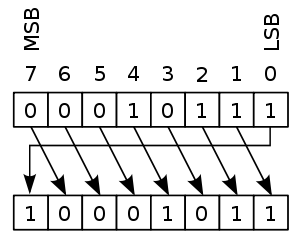
In coding theory, a cyclic code is a block code, where the circular shifts of each codeword gives another word that belongs to the code. They are error-correcting codes that have algebraic properties that are convenient for efficient error detection and correction.
If 00010111 is a valid codeword, applying a right circular shift gives the string 10001011. If the code is cyclic, then 10001011 is again a valid codeword. In general, applying a right circular shift moves the least significant bit (LSB) to the leftmost position, so that it becomes the most significant bit (MSB); the other positions are shifted by 1 to the right.
Definition[edit]
Let 









Cyclic codes have some additional structural constraint on the codes. They are based on Galois fields and because of their structural properties they are very useful for error controls. Their structure is strongly related to Galois fields because of which the encoding and decoding algorithms for cyclic codes are computationally efficient.
Algebraic structure[edit]
Cyclic codes can be linked to ideals in certain rings. Let ![R=A[x]/(x^{n}-1)](https://wikimedia.org/api/rest_v1/media/math/render/svg/8595f12ff1f64bc73a4e3e03851397cc877af6e2)











This must be a divisor of 
If the generator polynomial 



The idempotent of 









An irreducible code is a cyclic code in which the code, as an ideal is irreducible, i.e. is minimal in 
Examples[edit]
For example, if 


.
It corresponds to the ideal in ![mathbb {F} _{2}[x]/(x^{3}-1)](https://wikimedia.org/api/rest_v1/media/math/render/svg/9b419295ea611763fd442a22f8f56fe8dc30c023)

The polynomial 
The idempotent of this code is the polynomial 

Trivial examples[edit]
Trivial examples of cyclic codes are 



Over 



Quasi-cyclic codes and shortened codes[edit]
Before delving into the details of cyclic codes first we will discuss quasi-cyclic and shortened codes which are closely related to the cyclic codes and they all can be converted into each other.
Definition[edit]
Quasi-cyclic codes:[citation needed]
An 




Here, codeword polynomial is an element of a linear code whose code words are polynomials that are divisible by a polynomial of shorter length called the generator polynomial. Every codeword polynomial can be expressed in the form 






Definition[edit]
Shortened codes:
An ![[n,k]](https://wikimedia.org/api/rest_v1/media/math/render/svg/4d9e3e5f03db243c10e64608605d156ae1885952)


In shortened codes information symbols are deleted to obtain a desired blocklength smaller than the design blocklength. The missing information symbols are usually imagined to be at the beginning of the codeword and are considered to be 0. Therefore, 



All the symbols which are dropped need not be transmitted and at the receiving end can be reinserted. To convert 





Cyclic codes for correcting errors[edit]
Now, we will begin the discussion of cyclic codes explicitly with error detection and correction. Cyclic codes can be used to correct errors, like Hamming codes as cyclic codes can be used for correcting single error. Likewise, they are also used to correct double errors and burst errors. All types of error corrections are covered briefly in the further subsections.
The (7,4) Hamming code has a generator polynomial 









The received word is a polynomial of degree 

where 
We define the Syndrome Polynomial, 




For correcting two errors[edit]
Let the field elements 


Let 

These field elements are called «syndromes». Now because 






And these two can be considered as two pair of equations in 


Hence if the two pair of nonlinear equations can be solved cyclic codes can used to correct two errors.
Hamming code[edit]
The Hamming(7,4) code may be written as a cyclic code over GF(2) with generator 

![[2^{r}-1,2^{r}-r-2,4]](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbf755cb5b384b44e604aa2cc03660048b528348)
Hamming code for correcting single errors[edit]
A code whose minimum distance is at least 3, have a check matrix all of whose columns are distinct and non zero. If a check matrix for a binary code has 






It is easy to define Hamming codes for large alphabets of size 



So, there are 
![[(q^{m}-1)/(q-1),(q^{m}-1)/(q-1)-m]](https://wikimedia.org/api/rest_v1/media/math/render/svg/6447e6a6ef3646859efcaa879911eae094aae869)
Now, for cyclic codes, Let 






But for 



where, 


But we can also use 












Cyclic codes for correcting burst errors[edit]
From Hamming distance concept, a code with minimum distance 

A cyclic burst of length 

In polynomial form cyclic burst of length 








A linear block code that corrects all burst errors of length 











This property is also known as Rieger bound and it is similar to the Singleton bound for random error correcting.
Fire codes as cyclic bounds[edit]
In 1959, Philip Fire[6] presented a construction of cyclic codes generated by a product of a binomial and a primitive polynomial. The binomial has the form 



where 







A fire code can correct all burst errors of length t or less if no two bursts 







This shows that 


for some 






Since 















Fire codes are the best single burst correcting codes with high rate and they are constructed analytically. They are of very high rate and when 


For error detection cyclic codes are widely used and are called 
Cyclic codes on Fourier transform[edit]
Applications of Fourier transform are widespread in signal processing. But their applications are not limited to the complex fields only; Fourier transforms also exist in the Galois field 
Fourier transform over finite fields[edit]
Fourier transform over finite fields
The discrete Fourier transform of vector 




where exp(




If 









Here 











In Galois field time domain vector 



Spectral description of cyclic codes[edit]
Any codeword of cyclic code of blocklength 











Thus, cyclic codes can also be defined as
Given a set of spectral indices, 





So, cyclic codes are vectors in the field 



Following are the few bounds on the spectrum of cyclic codes.
BCH bound[edit]
If 





Hartmann-Tzeng bound[edit]
If 







components 



Roos bound[edit]
If 











Quadratic residue codes[edit]
When the prime 





Generalizations[edit]
A constacyclic code is a linear code with the property that for some constant λ if (c1,c2,…,cn) is a codeword then so is (λcn,c1,…,cn-1). A negacyclic code is a constacyclic code with λ=-1.[8] A quasi-cyclic code has the property that for some s, any cyclic shift of a codeword by s places is again a codeword.[9] A double circulant code is a quasi-cyclic code of even length with s=2.[9] Quasi-twisted codes and multi-twisted codes are further generalizations of constacyclic codes.[10][11]
See also[edit]
- Cyclic redundancy check
- BCH code
- Reed–Muller code
- Binary Golay code
- Ternary Golay code
- Eugene Prange
Notes[edit]
- ^ Van Lint 1998, p. 76
- ^ Van Lint 1998, p. 80
- ^ Hill 1988, pp. 159–160
- ^ Blahut 2003, Theorem 5.5.1
- ^ Hill 1988, pp. 162–163
- ^ P. Fire, E, P. (1959). A class of multiple-error-correcting binary codes for non-independent errors. Sylvania Reconnaissance Systems Laboratory, Mountain View, CA, Rept. RSL-E-2, 1959.
- ^ Wei Zhou, Shu Lin, Khaled Abdel-Ghaffar. Burst or random error correction based on Fire and BCH codes. ITA 2014: 1-5 2013.
- ^ Van Lint 1998, p. 75
- ^ a b MacWilliams & Sloane 1977, p. 506
- ^ Aydin, Nuh; Siap, Irfan; K. Ray-Chaudhuri, Dijen (2001). «The Structure of 1-Generator Quasi-Twisted Codes and New Linear Codes». Designs, Codes and Cryptography. 24 (3): 313–326. doi:10.1023/A:1011283523000. S2CID 17376783.
- ^ Aydin, Nuh; Halilović, Ajdin (2017). «A generalization of quasi-twisted codes: multi-twisted codes». Finite Fields and Their Applications. 45: 96–106. doi:10.1016/j.ffa.2016.12.002. S2CID 7694655.
References[edit]
- Blahut, Richard E. (2003), Algebraic Codes for Data Transmission (2nd ed.), Cambridge University Press, ISBN 0-521-55374-1
- Hill, Raymond (1988), A First Course In Coding Theory, Oxford University Press, ISBN 0-19-853803-0
- MacWilliams, F. J.; Sloane, N. J. A. (1977), The Theory of Error-Correcting Codes, New York: North-Holland Publishing, ISBN 0-444-85011-2
- Van Lint, J. H. (1998), Introduction to Coding Theory, Graduate Texts in Mathematics 86 (3rd ed.), Springer Verlag, ISBN 3-540-64133-5
Further reading[edit]
- Ranjan Bose, Information theory, coding and cryptography, ISBN 0-07-048297-7
- Irving S. Reed and Xuemin Chen, Error-Control Coding for Data Networks, Boston: Kluwer Academic Publishers, 1999, ISBN 0-7923-8528-4.
- Scott A. Vanstone, Paul C. Van Oorschot, An introduction to error correcting codes with applications, ISBN 0-7923-9017-2
External links[edit]
- John Gill’s (Stanford) class notes – Notes #3, October 8, Handout #9, EE 387.
- Jonathan Hall’s (MSU) class notes – Chapter 8. Cyclic codes — pp. 100 — 123
- David Terr. «Cyclic Code». MathWorld.
This article incorporates material from cyclic code on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.
In coding theory, a cyclic code is a block code, where the circular shifts of each codeword gives another word that belongs to the code. They are error-correcting codes that have algebraic properties that are convenient for efficient error detection and correction.
If 00010111 is a valid codeword, applying a right circular shift gives the string 10001011. If the code is cyclic, then 10001011 is again a valid codeword. In general, applying a right circular shift moves the least significant bit (LSB) to the leftmost position, so that it becomes the most significant bit (MSB); the other positions are shifted by 1 to the right.
Definition[edit]
Let 









Cyclic codes have some additional structural constraint on the codes. They are based on Galois fields and because of their structural properties they are very useful for error controls. Their structure is strongly related to Galois fields because of which the encoding and decoding algorithms for cyclic codes are computationally efficient.
Algebraic structure[edit]
Cyclic codes can be linked to ideals in certain rings. Let ![R=A[x]/(x^{n}-1)](https://wikimedia.org/api/rest_v1/media/math/render/svg/8595f12ff1f64bc73a4e3e03851397cc877af6e2)











This must be a divisor of 
If the generator polynomial 



The idempotent of 









An irreducible code is a cyclic code in which the code, as an ideal is irreducible, i.e. is minimal in 
Examples[edit]
For example, if 


.
It corresponds to the ideal in ![mathbb {F} _{2}[x]/(x^{3}-1)](https://wikimedia.org/api/rest_v1/media/math/render/svg/9b419295ea611763fd442a22f8f56fe8dc30c023)

The polynomial 
The idempotent of this code is the polynomial 

Trivial examples[edit]
Trivial examples of cyclic codes are 



Over 



Quasi-cyclic codes and shortened codes[edit]
Before delving into the details of cyclic codes first we will discuss quasi-cyclic and shortened codes which are closely related to the cyclic codes and they all can be converted into each other.
Definition[edit]
Quasi-cyclic codes:[citation needed]
An 




Here, codeword polynomial is an element of a linear code whose code words are polynomials that are divisible by a polynomial of shorter length called the generator polynomial. Every codeword polynomial can be expressed in the form 






Definition[edit]
Shortened codes:
An ![[n,k]](https://wikimedia.org/api/rest_v1/media/math/render/svg/4d9e3e5f03db243c10e64608605d156ae1885952)


In shortened codes information symbols are deleted to obtain a desired blocklength smaller than the design blocklength. The missing information symbols are usually imagined to be at the beginning of the codeword and are considered to be 0. Therefore, 



All the symbols which are dropped need not be transmitted and at the receiving end can be reinserted. To convert 





Cyclic codes for correcting errors[edit]
Now, we will begin the discussion of cyclic codes explicitly with error detection and correction. Cyclic codes can be used to correct errors, like Hamming codes as cyclic codes can be used for correcting single error. Likewise, they are also used to correct double errors and burst errors. All types of error corrections are covered briefly in the further subsections.
The (7,4) Hamming code has a generator polynomial 









The received word is a polynomial of degree 

where 
We define the Syndrome Polynomial, 




For correcting two errors[edit]
Let the field elements 


Let 

These field elements are called «syndromes». Now because 






And these two can be considered as two pair of equations in 


Hence if the two pair of nonlinear equations can be solved cyclic codes can used to correct two errors.
Hamming code[edit]
The Hamming(7,4) code may be written as a cyclic code over GF(2) with generator 

![[2^{r}-1,2^{r}-r-2,4]](https://wikimedia.org/api/rest_v1/media/math/render/svg/cbf755cb5b384b44e604aa2cc03660048b528348)
Hamming code for correcting single errors[edit]
A code whose minimum distance is at least 3, have a check matrix all of whose columns are distinct and non zero. If a check matrix for a binary code has 






It is easy to define Hamming codes for large alphabets of size 



So, there are 
![[(q^{m}-1)/(q-1),(q^{m}-1)/(q-1)-m]](https://wikimedia.org/api/rest_v1/media/math/render/svg/6447e6a6ef3646859efcaa879911eae094aae869)
Now, for cyclic codes, Let 






But for 



where, 


But we can also use 












Cyclic codes for correcting burst errors[edit]
From Hamming distance concept, a code with minimum distance 

A cyclic burst of length 

In polynomial form cyclic burst of length 








A linear block code that corrects all burst errors of length 











This property is also known as Rieger bound and it is similar to the Singleton bound for random error correcting.
Fire codes as cyclic bounds[edit]
In 1959, Philip Fire[6] presented a construction of cyclic codes generated by a product of a binomial and a primitive polynomial. The binomial has the form 



where 







A fire code can correct all burst errors of length t or less if no two bursts 







This shows that 


for some 






Since 















Fire codes are the best single burst correcting codes with high rate and they are constructed analytically. They are of very high rate and when 


For error detection cyclic codes are widely used and are called 
Cyclic codes on Fourier transform[edit]
Applications of Fourier transform are widespread in signal processing. But their applications are not limited to the complex fields only; Fourier transforms also exist in the Galois field 
Fourier transform over finite fields[edit]
Fourier transform over finite fields
The discrete Fourier transform of vector 




where exp(




If 









Here 











In Galois field time domain vector 



Spectral description of cyclic codes[edit]
Any codeword of cyclic code of blocklength 











Thus, cyclic codes can also be defined as
Given a set of spectral indices, 





So, cyclic codes are vectors in the field 



Following are the few bounds on the spectrum of cyclic codes.
BCH bound[edit]
If 





Hartmann-Tzeng bound[edit]
If 







components 



Roos bound[edit]
If 











Quadratic residue codes[edit]
When the prime 





Generalizations[edit]
A constacyclic code is a linear code with the property that for some constant λ if (c1,c2,…,cn) is a codeword then so is (λcn,c1,…,cn-1). A negacyclic code is a constacyclic code with λ=-1.[8] A quasi-cyclic code has the property that for some s, any cyclic shift of a codeword by s places is again a codeword.[9] A double circulant code is a quasi-cyclic code of even length with s=2.[9] Quasi-twisted codes and multi-twisted codes are further generalizations of constacyclic codes.[10][11]
See also[edit]
- Cyclic redundancy check
- BCH code
- Reed–Muller code
- Binary Golay code
- Ternary Golay code
- Eugene Prange
Notes[edit]
- ^ Van Lint 1998, p. 76
- ^ Van Lint 1998, p. 80
- ^ Hill 1988, pp. 159–160
- ^ Blahut 2003, Theorem 5.5.1
- ^ Hill 1988, pp. 162–163
- ^ P. Fire, E, P. (1959). A class of multiple-error-correcting binary codes for non-independent errors. Sylvania Reconnaissance Systems Laboratory, Mountain View, CA, Rept. RSL-E-2, 1959.
- ^ Wei Zhou, Shu Lin, Khaled Abdel-Ghaffar. Burst or random error correction based on Fire and BCH codes. ITA 2014: 1-5 2013.
- ^ Van Lint 1998, p. 75
- ^ a b MacWilliams & Sloane 1977, p. 506
- ^ Aydin, Nuh; Siap, Irfan; K. Ray-Chaudhuri, Dijen (2001). «The Structure of 1-Generator Quasi-Twisted Codes and New Linear Codes». Designs, Codes and Cryptography. 24 (3): 313–326. doi:10.1023/A:1011283523000. S2CID 17376783.
- ^ Aydin, Nuh; Halilović, Ajdin (2017). «A generalization of quasi-twisted codes: multi-twisted codes». Finite Fields and Their Applications. 45: 96–106. doi:10.1016/j.ffa.2016.12.002. S2CID 7694655.
References[edit]
- Blahut, Richard E. (2003), Algebraic Codes for Data Transmission (2nd ed.), Cambridge University Press, ISBN 0-521-55374-1
- Hill, Raymond (1988), A First Course In Coding Theory, Oxford University Press, ISBN 0-19-853803-0
- MacWilliams, F. J.; Sloane, N. J. A. (1977), The Theory of Error-Correcting Codes, New York: North-Holland Publishing, ISBN 0-444-85011-2
- Van Lint, J. H. (1998), Introduction to Coding Theory, Graduate Texts in Mathematics 86 (3rd ed.), Springer Verlag, ISBN 3-540-64133-5
Further reading[edit]
- Ranjan Bose, Information theory, coding and cryptography, ISBN 0-07-048297-7
- Irving S. Reed and Xuemin Chen, Error-Control Coding for Data Networks, Boston: Kluwer Academic Publishers, 1999, ISBN 0-7923-8528-4.
- Scott A. Vanstone, Paul C. Van Oorschot, An introduction to error correcting codes with applications, ISBN 0-7923-9017-2
External links[edit]
- John Gill’s (Stanford) class notes – Notes #3, October 8, Handout #9, EE 387.
- Jonathan Hall’s (MSU) class notes – Chapter 8. Cyclic codes — pp. 100 — 123
- David Terr. «Cyclic Code». MathWorld.
This article incorporates material from cyclic code on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.
Основной недостаток применения групповых
линейных кодов – сложность кодирующих
и декодирующих устройств, являющихся
устройствами параллельного типа. Переход
к циклическим кодам позволяет упростить
схемную реализацию кодирующих и
декодирующих устройств за счет
многотактных способов обработки
циклических кодов с помощью сдвиговых
регистров. Таким образом, в системах,
использующих циклические коды,
«обменивают» увеличение времени
кодирования и декодирования на упрощение
аппаратуры. Циклическим кодом называют
такой групповой код, который замкнут
относительно циклической перестановки
любой кодовой комбинации этого кода,
т.е. в результате циклической перестановки
любого кодового вектора получается
кодовый вектор, принадлежащий этому же
циклическому коду.
Циклической перестановкой называется
такая перестановка, при которой все
разряды смещаются в сторону старших
разрядов, причем последний разряд
переходит на место первого.
1.2 Построение цк
Циклическим кодом называется циклического
векторное пространство, обладающее
всеми свойствами группового кода, но
замкнутого не только относительно
линейных операций, но и относительно
операции циклического сдвига. В результате
циклического сдвига любого кодового
вектора получается кодовый вектор,
принадлежащий этому же векторному
пространству. В основе циклических
кодов лежит алгебра полиномов по модулю
над
пространственным полем (полем Галуа).
Циклическим сдвигом называется такой
сдвиг, при котором все разряды смещаются
в сторону старших разрядов, причем
последний разряд переходит на место
первого.
Пусть
Тогда после циклического сдвига получим
Математической основой построения
циклических кодов является представление
любого двоичного числа в виде полинома,
содержащего переменную Х, причем двоичные
цифры являются коэффициентами при этой
переменной.
Пример 1
В дальнейшем, если нет особых замечаний,
высшие степени пишем справа. Над такими
полиномами можно производить все
операции согласно законам алгебры.
Однако при всех операциях суммирование
производиться по модулю 2 (без переносов
в старшие разряды в отличие от
арифметического суммирования).
Вычитание, используемое при делении
полиномов, также необходимо осуществлять
по модулю два (mod2), и оно
заменяется эквивалентной операцией
суммирования поmod2.
Пример 2
Циклический код полностью задаётся
так называемым порождающим полиномом
g(x) степениk, гдеk–
число контрольных (избыточных) символов
циклического кода.
Кодовые комбинации циклического кода
строятся так, чтобы соответствующие им
полиномы делились без остатка на g(x).
С другой стороны, циклический код может
быть полностью определен многочленомh(x) степениm, гдеm–
число информационных символов циклического
кода:
(1)
n– число разрядов (длина)
циклического кода (n=m+k):
Полином, соответствующий кодовой
комбинации циклического кода, имеет
вид:
Циклические коды, исправляющие одну
ошибку (),
либо исправляющие одну и обнаруживающие
две ошибки ()
называются циклическими кодами Хемминга.
Для циклических кодов Хемминга имеет
место
Пример 3
Наибольший интерес представляют
систематические циклические коды, так
как они позволяют наиболее просто
реализовать кодирующие и декодирующие
устройства. Условимся в любом
систематическом циклическом коде первые
символов, т.е. коэффициенты при
всегда
выбирать в качестве проверочных символов,
а последниеmсимволов,
т.е. коэффициенты при— в качестве информационных символов.
Соседние файлы в папке Теория
- #
- #
- #
- #
- #
03.07.20181.15 Mб3Теория.mht
- #
4. Циклические коды
4.1 Основные понятия
Циклическим кодом
называется линейный блоковый (n,k)-код, который характеризуется свойством
цикличности, т.е. сдвиг влево на один шаг любого разрешенного кодового слова
дает также разрешенное кодовое слово, принадлежащее этому же коду и у которого,
множество кодовых слов представляется совокупностью многочленов степени (n-1) и
менее, делящихся на некоторый многочлен g(x) степени r = n-k, являющийся
сомножителем двучлена xn+1.
Многочлен g(x) называется
порождающим.
Как следует из определения, в циклическом коде кодовые слова
представляются в виде многочленов 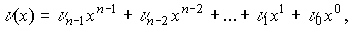
где n — длина кода;
— коэффициенты из поля GF(q).
Если код построен над полем GF(2), то
коэффициенты принимают значения 0 или 1 и код называется двоичным.
Пример. Если кодовое слово циклического кода 
то соответствующий
ему многочлен 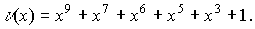
Например, если код
построен над полем GF(q)=GF(23), которое является расширением GF(2)
по модулю неприводимого многочлена f(z)=z3+z+1, а элементы этого поля
имеют вид, представленный в таблице 3,
| 0 | 000 | 0 | a3 | 011 | Z+1 |
| a0 | 001 | 1 | a4 | 110 | Z2+Z |
| a1 | 010 | Z | a5 | 111 | Z2+Z+1 |
| a2 | 100 | Z2 | a6 | 101 | Z2+1 |
то
коэффициенты 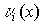
элементов этого поля и поэтому они сами отображаются в виде многочленов
следующего вида
где m — степень многочлена, по которому получено расширение поля GF(2);
ai — коэффициенты, принимающие значение элементов GF(2), т.е. 0 и
1.
Такой код называется q-ным.
Длина циклического кода
называется примитивной и сам код называется примитивным, если его длина
n=qm-1 над GF(q).
Если длина кода меньше длины примитивного кода,
то код называется укороченным или непримитивным.
Как следует
из определения общее свойство кодовых слов циклического кода — это их делимость
без остатка на некоторый многочлен g(x), называемый порождающим.
Результатом деления двучлена xn+1 на многочлен g(x)
является проверочный многочлен h(x).
При декодировании
циклических кодов используются многочлен ошибок e(x) и синдромный многочлен
S(x).
Многочлен ошибок степени не более (n-1) определяется из выражения
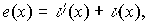
где 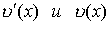
многочлены, отображающие соответственно принятое (с ошибкой) и переданное
кодовые слова.
Ненулевые коэффициенты в е(x) занимают позиции, которые
соответствуют ошибкам.
Пример.
Синдромный многочлен, используемый при декодировании циклического
кода, определяется как остаток от деления принятого кодового слова на
порождающий многочлен, т.е. 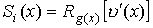
или
Следовательно, синдромный многочлен зависит непосредственно от многочлена
ошибок е(х).Это положение используется при построении таблицы
синдромов, применяемой в процессе декодирования. Эта таблица содержит список
многочленов ошибок (см. первый столбец стандартного расположения кода в разделе
2.4) и список соответствующих синдромов, определяемых из выражения 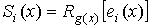
таблицу 4).
| (x) | S(x) |
| 1 | Rg(x)[1] |
| X | Rg(x)[x] |
| X2 | Rg(x)[x2] |
| · | · |
| · | · |
| · | · |
| X+1 | Rg(x)[x+1] |
| X2+1 | Rg(x)[x2+1] |
| · | · |
| · | · |
| · | · |
В процессе
декодирования по принятому кодовому слову вычисляется синдром, затем в таблице
находится соответствующий многочлен е(х), суммирование которого с принятым
кодовым словом дает исправленное кодовое слово, т.е. 
Перечисленные
многочлены 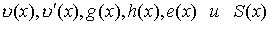
умножать и делить, используя известные правила алгебры, но с приведением
результата по mod 2, а затем по mod xn+1, если степень результата
превышает степень (n-1).
Примеры.
Допустим,
что длина кода n=7, то результат приводим по mod x7+1.
При
построении и декодировании циклических кодов в результате деления многочленов
обычно необходимо иметь не частное, а остаток от деления.
Поэтому
рекомендуется более простой способ деления, используя не многочлены, а только
его коэффициенты (вариант 2 в примере).
- Пример.
- 1.
- 2.
4.2 Матричное задание кодов
Циклический
код может быть задан порождающей и проверочной матрицами. Для их построения
достаточно знать порождающий g(x) и проверочный h(x) многочлены.
Для несистематического циклического кода матрицы строятся
циклическим сдвигом порождающего и проверочного многочленов, т.е. путем их
умножения на x 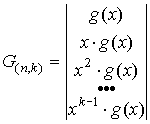
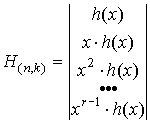
При построении
матрицы H(n,k) старший коэффициент многочлена h(x) располагается
справа.
Пример. Для циклического (7,4)-кода с порождающим многочленом
g(x)=x3+x+1 матрицы G(n,k) и H(n,k) имеют вид:

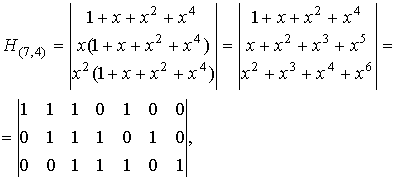
где 
Для систематического циклического кода матрица G(n,k)
определяется из выражения 
где
Ik — единичная матрица;
Rk,r — прямоугольная
матрица.
Строки матрицы Rk,r определяются из выражений 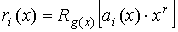
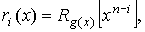
где
ai(x) — значение i-той строки матрицы Ik;
i — номер
строки матрицы Rk,r.
Пример. Матрица G(n,k) для
(7,4)-кода на основе порождающего многочлена g(x)=x3+x+1, строится в
следующей последовательности 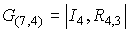
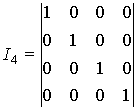
Определяется
R4,3, используя 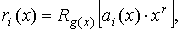
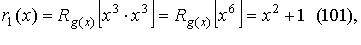


Аналогичным способом
определяется
В результате получаем 
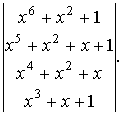
Используя выражение
получим тот же результат.
Строки матрицы G(n,k) можно определить
непосредственно из выражения 
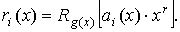
Проверочная матрица в систематическом виде строится на основе
матрицы G(n,k), а именно: 
где
Ir — единичная матрица; 
G(n,k) в транспонированном виде.
Пример. Для (7,4)-кода
матрица H(n,k) будет иметь вид: 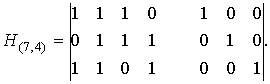
Одна из основных
задач, стоящих перед разработчиками устройств защиты от ошибок при передаче
дискретных сообщений по каналам связи является выбор порождающего многочлена
g(x) для построения циклического кода, обеспечивающего требуемое минимальное
кодовое расстояние для гарантийного обнаружения и исправления t-кратных ошибок.
Существуют специальные таблицы по выбору g(x) в зависимости от предъявляемых
требований к корректирующим возможностям кода. Однако у каждого циклического
кода имеются свои особенности формирования g(x). Поэтому при изучении конкретных
циклических кодов будут рассматриваться соответствующие способы построения g(x).
4.3 Коды БЧХ
Одним из классов
циклических кодов, способных исправлять многократные ошибки, являются коды БЧХ.
Примитивным кодом БЧХ, исправляющим tu ошибок,
называется код длиной n=qm-1 над GF(q), для которого элементы 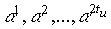
корнями порождающего многочлена.
Здесь a —
примитивный элемент GF(qm).
Порождающий многочлен
определяется из выражения 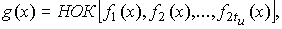
f1(x),f2(x)…- минимальные многочлены корней g(x).
Число проверочных элементов кода БЧХ удовлетворяет соотношению
Пример. Определить значение порождающего многочлена для построения
примитивного кода БЧХ над GF(2) длины 31, исправляющего двух кратные ошибки
(tu=2).
Исходя из определения кода БЧХ корнями многочлена g(x)
являются: 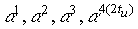
Порождающий многочлен определяется из выражения 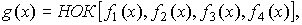
f2(x), f3(x), f4(x) — минимальные многочлены
корней соответственно 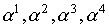
Примечание.
Минимальный многочлен элемента b поля
GF(qm) определяется из выражения 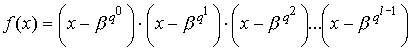

число, при котором 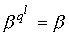
Значения
минимальных многочленов будут следующими: 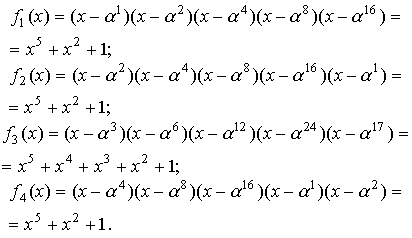
Так как f1(x)=
f2(x)= f4(x), то 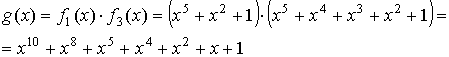
На
практике при определении значений порождающего многочлена пользуются специальной
таблицей минимальных многочленов (см. таблицу 8 приложения), и выражением для
порождающего многочлена 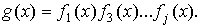
осуществляется в следующей последовательности.
По заданной длине кода n и
кратности исправляемых ошибок tu определяют:
— из выражения
n=2m-1 значение параметра m, который является максимальной степенью
сомножителей g(x);
— из выражения j=2tu-1 максимальный порядок
минимального многочлена, входящего в число сомножителей g(x).
— пользуясь
таблицей минимальных многочленов, определяется выражение для g(x) в зависимости
от m и j. Для этого из колонки, соответствующей параметру m, выбираются
многочлены с порядками от 1 до j, которые в результате перемножения дают
значение g(x).
Примечание.
В выражении для g(x) содержаться минимальные
многочлены только для нечетных степеней a, так как
обычно соответствующие им минимальные многочлены четных степеней a имеют аналогичные выражения.
Например, минимальные
многочлены элементов 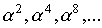
минимальному многочлену элемента a1,
минимальные многочлены элементов 
минимальному многочлену a3 и т.п.
Пример. Определить значение порождающего многочлена для построения
примитивного кода БЧХ над GF(2) длины 31, обеспечивающего tu=3.
Определяем значения m и j. 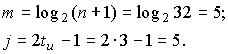
Из таблицы
минимальных многочленов в соответствии с m=5 и j=5 получаем 
Заданные исходные
данные: n и tu или k и tu для построения циклического кода
часто приводят к выбору завышенного значения m и как следствие этого к
неоправданному увеличению длины кода. Такое положение снижает эффективность
полученного кода, так как часть информационных разрядов вообще не используется.
Пример. Требуется построить циклический код, исправляющий двух
кратные ошибки, если длина информационной части кода k=40.
Согласно
выражению для примитивного кода n=2m-1, ближайшая длина кода равна
63, для которой m=6, а r=mtu=12. Следовательно, код будет иметь n=63,
k=51. Неиспользованных информационных разрядов будет 11(51-40).
Подобное
несоответствие в ряде случаев можно устранить, применяя непримитивный код БЧХ.
Непримитивным кодом БЧХ, исправляющим tu ошибок,
называется код длины n над GF(q), для которого элементы 
порождающего многочлена.
Здесь bi-непримитивный элемент GF(qm), а
длина кода n равна порядку элемента bi.
Примечание.
Порядком элемента bi
является наименьшее n, для которого 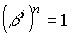
Пример.
Порядок элемента b3 поля GF(26)
равен 21, так как 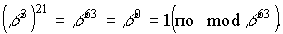
Порождающий многочлен непримитивного кода БЧХ, по аналогии с
примитивным кодом, определяется из выражения 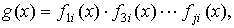
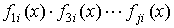
элементов 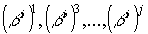
GF(qm), которые являются корнями g(x); i — степень непримитивного
элемента b.
Пример. Определить значение g(x)
для построения непримитивного кода БЧХ над GF(2) длины n=20, исправляющего двух
кратные ошибки.
Из таблицы непримитивных элементов GF(2m) (см.
таблицу 7 приложения) выбираем поле, элемент b которого
имеет порядок больший, но близкий к заданному n.
Такими являются
GF(26) и b3, порядок которого
n=21.
Так как j=2tu-1=2(2-1=3, то выражение для g(x) будет иметь
вид
где f3(x) и f9(x) — минимальные многочлены элементов
b3 и b9
поля GF(26).
Значения этих многочленов следующие: 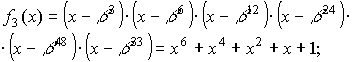
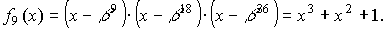
Выражения для
f3(x) и f9(x) можно определить из таблицы минимальных
многочленов, используя для этого параметр m выбранного поля GF(2m) и
порядковые номера сомножителей g(x).
Для рассмотренного примера m=6, а
порядковые номера равны 3 и 9. Поэтому 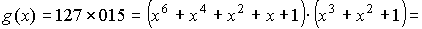
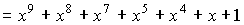
4.4 Способы кодирования
Задача кодирования
заключается в формировании по информационным словам a(x) кодовых слов u(x) циклического (n,k)-кода, который по своей структуре
может быть несистематическим и систематическим.
Формирование
кодовых слов несистематического кода заключается в умножении многочлена a(x),
отображающего информационную последовательность длины k, на порождающий
многочлен, т.е. u(x)=a(x)(g(x). Формирование кодовых
слов систематического кода заключается в преобразовании информационной
последовательности a(x) в соответствии с выражением u(x)=a(x)·xr+r(x).
Проверочная последовательность r(x) определяется двумя способами:
при использовании «классического» способа кодирования 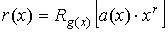
при использовании
способа кодирования, рекомендованного МККТТ 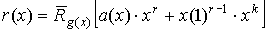
где
x(1)r-1 — единичный многочлен степени (r-1).
Указанные выше
математические операции выполняют кодеры несистематического и систематического
кодов.
4.5 Способы декодирования с обнаружением ошибок
Процедура декодирования
циклического кода с обнаружением ошибок, по аналогии с процессом кодирования,
использует два способа:
— при кодировании «классическим»
способом декодирование основано на использовании свойства делимости без остатка
кодового многочлена u(x) циклического (n,k)-кода на
порождающий многочлен g(x). Поэтому алгоритм декодирования включает в себя
деление принятого кодового слова, описываемого многочленом 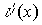
анализ остатка r(x). Если r(x)=0, то принятое кодовое слово считается
неискаженным. Если r(x)№0, то принятое кодовое слово
стирается и формируется сигнал «ошибка».
— при кодировании
способом МККТТ декодирование основано на свойстве получения определенного
контрольного остатка R0(x) при делении принятого кодового многочлена
u(x) на порождающий многочлен. Поэтому, если полученный
при делении остаток 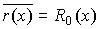
слово считается неискаженным. Если остаток 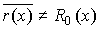
слово стирается и формируется сигнал «ошибка».
Значение контрольного остатка
определяется из выражения 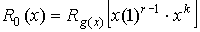
4.6 Способы декодирования с исправлением ошибок
и схемная реализация декодирующих устройств
и схемная реализация декодирующих устройств
Декодирование циклического
кода в режиме исправления ошибок можно осуществлять различными способами. Ниже
излагаются два способа, являющиеся наиболее простыми.
В
основу первого способа положено использование таблицы синдромов (декодирования),
в которой каждому многочлену или образцу ошибок ei(x), соответствует
определенный синдром Si(x), представляющий остаток от деления
принятого кодового слова 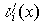
ei(x) на g(x). Процедура декодирования следующая. Принятое кодовое
слово 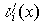
определяется Si(x) и соответствующий ему многочлен ei(x),
а затем 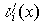
ei(x). В результате получаем исправленное кодовое слово, т.е. 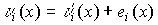
В состав декодера входят: вычислитель
синдрома (ВС), два регистра сдвига RG1 и RG2, постоянное запоминающее устройство
(ПЗУ), котороесодержит 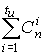
соответствующие многочленам ошибок ei(x).
Принятое кодовое слово
поступает на вход вычислителя синдрома, где осуществляется деление его на g(x) и
формирование Si(x), и одновременно — на вход RG2, где
накапливается. Синдром Si(x) используется в качестве адреса, по
которому из ПЗУ в регистр RG1 записывается ei(x), соответствующий
синдрому Si(x). Перечисленные операции завершаются за n тактов. В
течение последующих n тактов происходит поэлементное суммирование содержимого
RG2 и RG1, т.е. операция 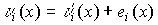
В основе второго способа исправления ошибок, позволяющего
значительно сократить объем используемых табличных синдромов и существенно
упростить схему декодера, лежат следующие положения:
1. Синдром
Si(x), соответствующий принятому кодовому слову равен остатку от
деления 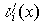
от деления соответствующего многочлена ошибок ei(x) на g(x), т.е. 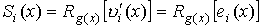
2.
Если Si(x) соответствует 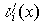
x( Si(x) является синдромом, который соответствует 

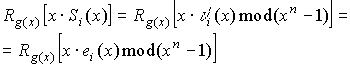
3.
При исправлении ошибок используются синдромы образцов ошибок только с ненулевым
коэффициентом в старшем разряде.
Поэтому при реализации
этого способа множество всех образцов ошибок разбивается на классы
эквивалентности. Каждый класс представляет циклический сдвиг одного образца
ошибок, а синдром этого класса соответствует образцу ошибок с ненулевым старшим
разрядом. Если вычисленный синдром принадлежит одному из классов эквивалентности
образцов исправляемых ошибок, то старший символ кодового слова исправляется.
Затем принятое слово и синдром циклически сдвигается, а процесс нахождения в
предыдущей по старшинству позиции повторяется.
Для исправления ошибок,
принадлежащих данному классу эквивалентности, нужно произвести n циклических
сдвигов.
Простейшим является декодер Меггитта. В состав декодера
входят: вычислитель синдрома, осуществляющий деление кодового слова 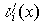
и формирование соответствующего синдрома; блок декодеров (ДК), который настроен
на синдромы всех образцов исправляемых ошибок с ненулевыми старшими разрядами;
регистр сдвига RG.
При поступлении на вход схемы кодового слова 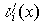
символы заполняют регистр RG, а в вычислителе формируется соответствующий
синдром Si(x). Вычисленный синдром сравнивается со всеми табличными
синдромами, заложенными в схему блока ДК, и в случае совпадения с одним из них
на его выходе формируется сигнал, который исправляет ошибочный символ,
находящийся в старшем разряде регистра. После этого содержимое вычислителя и RG
циклически сдвигается на один шаг. Этот сдвиг реализует операции 

новый синдром совпадает с одним из табличных синдромов, то это означает, что
произошла ошибка во втором по старшинству символе кодового слова, который,
перейдя в старший разряд RG, исправляется. Затем производится новый циклический
сдвиг на одну позицию и новая проверка на совпадение синдромов. После повторения
этого процесса n раз в RG будет сформировано исправленное кодовое слово.
Введение обратной связи для RG не обязательно, так как в процессе исправления
ошибок символы кодового слова поступают на выход декодера.
Пример. Рассмотрим схему и работу декодера Меггитта
циклического (15,7)-кода, обеспечивающего исправление одиночных и двойных
ошибок, с g(x)=x8+ x7+ x6+ x4+1 (см.
рисунок 8).
Блок декодеров настраивается на 15 синдромов, которые
представлены в таблице 5 и соответствуют классам эквивалентности с образцами
ошибок в старшем разряде.
| № | е(х) | S(x) | № | е(х) | S(x) |
|---|---|---|---|---|---|
| 1 | x14 | x7+ x6+x5+ x3 |
9 | x14+ x6 | |
| 2 | x14+ x13 | x7+ x4+x3+ x2 |
10 | x14+ x5 | x7+ x6+x3 |
| 3 | x14+ x12 | x7+ x6+x4+ x |
11 | x14+ x4 | x7+ x6+x5+ x4+x3 |
| 4 | x14+ x11 | 12 | x14+ x3 | x7+ x6+x5 |
|
| 5 | x14+ x10 | 13 | x14+ x2 | x7+ x6+x5+ x3+x2 |
|
| 6 | x14+ x9 | 14 | x14+ x1 | x7+ x6+x5+ x3+x |
|
| 7 | x14+ x8 | 15 | x14+ x0 | x7+ x6+x5+ x3+0 |
|
| 8 | x14+ x7 |
Допустим, что
ошибки в 3 и 5 разрядах, т.е. им соответствует многочлен ошибки
e(x)=x12+x10.
При поступлении на вход декодера
искаженного кодового слова он заполняет регистр и в вычислителе формируется
синдром 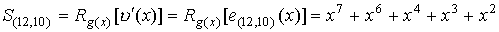
Блок декодеров не
реагирует на этот синдром.
Затем происходит сдвиг кодового слова в RG, а в
BC формируется новый синдром 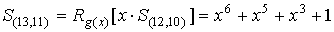
Блок декодеров и в
этом случае не срабатывает.
При следующем сдвиге кодового слова в RG первый
искаженный разряд занимает старшую позицию в RG, а в BC формируется синдром 
которого срабатывает БДК. В результате исправляется первая ошибка.
Следующим
сдвиг приводит к формированию синдрома 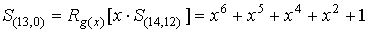
Этот синдром
соответствует многочлену ошибки e(x)=x13+x0, т.к. первый
искаженный разряд по обратной связи должен занять младшую позицию RG.
На
синдром S(13,0) блок декодеров не реагирует.
При следующем сдвиге
кодового слова в RG второй искаженный разряд занимает старшую позицию в RG, а в
BC формируется синдром 
БДК. В результате исправляется вторая ошибка в кодовом слове.
4.7 Коды Рида-Соломона (РС)
Коды РС
являются недвоичными циклическими кодами, символы кодовых слов которых берутся
из конечного поля GF(q). Здесь q степень некоторого простого числа, например
q=2m.
Допустим, что РС-код построен над GF(8), которое является
расширением поля GF(2) по модулю примитивного многочлена f(z)=z3+z+1.
В этом случае символы кодовых слов кода будут иметь значения, представленные в
таблице 6.
| 000 | 0 | 0 | 011 | z+1 | a3 |
| 001 | 1 | a0 | 110 | z2+z | a4 |
| 010 | z | a1 | 111 | z2+z+1 | a5 |
| 100 | z2 | a2 | 101 | z2+1 | a6 |
Кодовые слова РС-кода
отображаются в виде многочленов 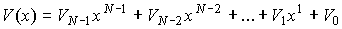
где N — длина кода;
Vi — q-ичные коэффициенты (символы кодовых слов), которые могут
принимать любое значение из GF(q).
Эти коэффициенты как это следует из
таблицы, также отображаются многочленами с двоичными коэффициентами 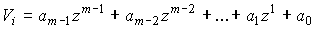
информационной последовательности k они обладают наибольшим кодовым расстоянием
d=N-k+1.
Порождающим многочленом g(x) РС-кода является делитель двучлена
xN+1 степени меньшей N с коэффициентами из GF(q) при условии, что
элементы 
корнями g(x). Здесь 
GF(q).
На основе этого определения, а также теоремы Безу,
выражение для порождающего многочлена РС-кода будет иметь вид 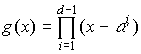
Степень g(x) равна d-1=N-k=R.
В РС-кодах принадлежность кодовых слов
данному коду определяется выполнением d-1 уравнений в соответствии с выражением
(*),
где Vi — символы-коэффициенты из GF(q);
z0,
z1… zN-1 — ненулевые элементы GF(q).
Элементы
z0, z1… zN-1 называются локаторами, т.е.
указывающими на номер позиции символа кодового слова.
Например, указателем i
— позиции является локатор zi или элемент ai
GF(q).
Так как все локаторы должны быть различны и причем ненулевыми, то их
число в GF(q) равно q-1. Следовательно, такое количество символов должно быть в
кодовых словах кода.Поэтому обычно длина РС-кода определяется из
выражения N=q-1.
Пример. Допустим, что длина РС-кода равна N, кодовое
расстояние d=3, то в соответствии с (*) проверочными уравнениями будут 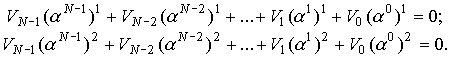
Свойства РС-кодов.
1. Циклический сдвиг кодовых слов, символы которых принимают значение из
GF(q), порождает новые кодовые слова этого же кода.
2. Сумма по mod2 двух и
более кодовых слов дает кодовое слово, принадлежащее этому же коду.
3.
Кодовое расстояние РС-кода определяется не по двоичным элементам, а по q-ичным
символам.
4. В РС-коде, исправляющем tu ошибок порождающий
многочлен определяется из выражения 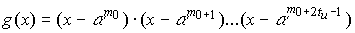
принимают равным 1. Однако, с помощью разумного выбора значения m0,
иногда можно упростить схему кодера.
5. Корректирующие способности РС-кода
определяются его кодовым расстоянием. 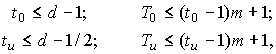
где T0 и
Tu — длина пакетов, в которых обнаруживаются и исправляются ошибки.
Обнаружение ошибок в кодовых словах состоит в проверке условий ((), т.е.
определении синдрома 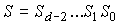
определяются из выражения 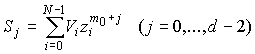
Пример. Требуется сформировать кодовое слово РС-кода над
GF(23), соответствующее двоичной информационной последовательности
a(1,0)=000000011100101.
Так как m=3, то каждый q-ичный символ кода состоит
из трех двоичных элементов. Поэтому с учетом таблицы 6 a(x)=a3x2+ a2x+a6.
Определяем параметры кода.
N=q-1=7; k=5; R=2; d=N-k+1=3; 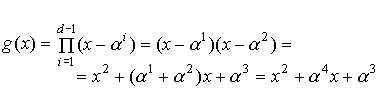
Кодовое слово формируется в соответствии с выражением. 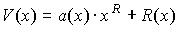
где
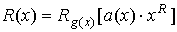
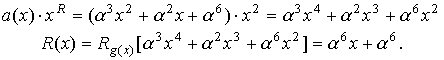
В
результате 
V(1,0)=000.000.011.100.101.101.101.