«Interleaver» redirects here. For the fiber-optic device, see optical interleaver.
In computing, telecommunication, information theory, and coding theory, forward error correction (FEC) or channel coding[1][2][3] is a technique used for controlling errors in data transmission over unreliable or noisy communication channels.
The central idea is that the sender encodes the message in a redundant way, most often by using an error correction code or error correcting code, (ECC).[4][5] The redundancy allows the receiver not only to detect errors that may occur anywhere in the message, but often to correct a limited number of errors. Therefore a reverse channel to request re-transmission may not be needed. The cost is a fixed, higher forward channel bandwidth.
The American mathematician Richard Hamming pioneered this field in the 1940s and invented the first error-correcting code in 1950: the Hamming (7,4) code.[5]
FEC can be applied in situations where re-transmissions are costly or impossible, such as one-way communication links or when transmitting to multiple receivers in multicast.
Long-latency connections also benefit; in the case of a satellite orbiting Uranus, retransmission due to errors can create a delay of five hours. FEC is widely used in modems and in cellular networks, as well.
FEC processing in a receiver may be applied to a digital bit stream or in the demodulation of a digitally modulated carrier. For the latter, FEC is an integral part of the initial analog-to-digital conversion in the receiver. The Viterbi decoder implements a soft-decision algorithm to demodulate digital data from an analog signal corrupted by noise. Many FEC decoders can also generate a bit-error rate (BER) signal which can be used as feedback to fine-tune the analog receiving electronics.
FEC information is added to mass storage (magnetic, optical and solid state/flash based) devices to enable recovery of corrupted data, and is used as ECC computer memory on systems that require special provisions for reliability.
The maximum proportion of errors or missing bits that can be corrected is determined by the design of the ECC, so different forward error correcting codes are suitable for different conditions. In general, a stronger code induces more redundancy that needs to be transmitted using the available bandwidth, which reduces the effective bit-rate while improving the received effective signal-to-noise ratio. The noisy-channel coding theorem of Claude Shannon can be used to compute the maximum achievable communication bandwidth for a given maximum acceptable error probability. This establishes bounds on the theoretical maximum information transfer rate of a channel with some given base noise level. However, the proof is not constructive, and hence gives no insight of how to build a capacity achieving code. After years of research, some advanced FEC systems like polar code[3] come very close to the theoretical maximum given by the Shannon channel capacity under the hypothesis of an infinite length frame.
How it works[edit]
ECC is accomplished by adding redundancy to the transmitted information using an algorithm. A redundant bit may be a complex function of many original information bits. The original information may or may not appear literally in the encoded output; codes that include the unmodified input in the output are systematic, while those that do not are non-systematic.
A simplistic example of ECC is to transmit each data bit 3 times, which is known as a (3,1) repetition code. Through a noisy channel, a receiver might see 8 versions of the output, see table below.
| Triplet received | Interpreted as |
|---|---|
| 000 | 0 (error-free) |
| 001 | 0 |
| 010 | 0 |
| 100 | 0 |
| 111 | 1 (error-free) |
| 110 | 1 |
| 101 | 1 |
| 011 | 1 |
This allows an error in any one of the three samples to be corrected by «majority vote», or «democratic voting». The correcting ability of this ECC is:
- Up to 1 bit of triplet in error, or
- up to 2 bits of triplet omitted (cases not shown in table).
Though simple to implement and widely used, this triple modular redundancy is a relatively inefficient ECC. Better ECC codes typically examine the last several tens or even the last several hundreds of previously received bits to determine how to decode the current small handful of bits (typically in groups of 2 to 8 bits).
Averaging noise to reduce errors[edit]
ECC could be said to work by «averaging noise»; since each data bit affects many transmitted symbols, the corruption of some symbols by noise usually allows the original user data to be extracted from the other, uncorrupted received symbols that also depend on the same user data.
- Because of this «risk-pooling» effect, digital communication systems that use ECC tend to work well above a certain minimum signal-to-noise ratio and not at all below it.
- This all-or-nothing tendency – the cliff effect – becomes more pronounced as stronger codes are used that more closely approach the theoretical Shannon limit.
- Interleaving ECC coded data can reduce the all or nothing properties of transmitted ECC codes when the channel errors tend to occur in bursts. However, this method has limits; it is best used on narrowband data.
Most telecommunication systems use a fixed channel code designed to tolerate the expected worst-case bit error rate, and then fail to work at all if the bit error rate is ever worse.
However, some systems adapt to the given channel error conditions: some instances of hybrid automatic repeat-request use a fixed ECC method as long as the ECC can handle the error rate, then switch to ARQ when the error rate gets too high;
adaptive modulation and coding uses a variety of ECC rates, adding more error-correction bits per packet when there are higher error rates in the channel, or taking them out when they are not needed.
Types of ECC[edit]
A block code (specifically a Hamming code) where redundant bits are added as a block to the end of the initial message
A continuous code convolutional code where redundant bits are added continuously into the structure of the code word
The two main categories of ECC codes are block codes and convolutional codes.
- Block codes work on fixed-size blocks (packets) of bits or symbols of predetermined size. Practical block codes can generally be hard-decoded in polynomial time to their block length.
- Convolutional codes work on bit or symbol streams of arbitrary length. They are most often soft decoded with the Viterbi algorithm, though other algorithms are sometimes used. Viterbi decoding allows asymptotically optimal decoding efficiency with increasing constraint length of the convolutional code, but at the expense of exponentially increasing complexity. A convolutional code that is terminated is also a ‘block code’ in that it encodes a block of input data, but the block size of a convolutional code is generally arbitrary, while block codes have a fixed size dictated by their algebraic characteristics. Types of termination for convolutional codes include «tail-biting» and «bit-flushing».
There are many types of block codes; Reed–Solomon coding is noteworthy for its widespread use in compact discs, DVDs, and hard disk drives. Other examples of classical block codes include Golay, BCH, Multidimensional parity, and Hamming codes.
Hamming ECC is commonly used to correct NAND flash memory errors.[6]
This provides single-bit error correction and 2-bit error detection.
Hamming codes are only suitable for more reliable single-level cell (SLC) NAND.
Denser multi-level cell (MLC) NAND may use multi-bit correcting ECC such as BCH or Reed–Solomon.[7][8] NOR Flash typically does not use any error correction.[7]
Classical block codes are usually decoded using hard-decision algorithms,[9] which means that for every input and output signal a hard decision is made whether it corresponds to a one or a zero bit. In contrast, convolutional codes are typically decoded using soft-decision algorithms like the Viterbi, MAP or BCJR algorithms, which process (discretized) analog signals, and which allow for much higher error-correction performance than hard-decision decoding.
Nearly all classical block codes apply the algebraic properties of finite fields. Hence classical block codes are often referred to as algebraic codes.
In contrast to classical block codes that often specify an error-detecting or error-correcting ability, many modern block codes such as LDPC codes lack such guarantees. Instead, modern codes are evaluated in terms of their bit error rates.
Most forward error correction codes correct only bit-flips, but not bit-insertions or bit-deletions.
In this setting, the Hamming distance is the appropriate way to measure the bit error rate.
A few forward error correction codes are designed to correct bit-insertions and bit-deletions, such as Marker Codes and Watermark Codes.
The Levenshtein distance is a more appropriate way to measure the bit error rate when using such codes.
[10]
Code-rate and the tradeoff between reliability and data rate[edit]
The fundamental principle of ECC is to add redundant bits in order to help the decoder to find out the true message that was encoded by the transmitter. The code-rate of a given ECC system is defined as the ratio between the number of information bits and the total number of bits (i.e., information plus redundancy bits) in a given communication package. The code-rate is hence a real number. A low code-rate close to zero implies a strong code that uses many redundant bits to achieve a good performance, while a large code-rate close to 1 implies a weak code.
The redundant bits that protect the information have to be transferred using the same communication resources that they are trying to protect. This causes a fundamental tradeoff between reliability and data rate.[11] In one extreme, a strong code (with low code-rate) can induce an important increase in the receiver SNR (signal-to-noise-ratio) decreasing the bit error rate, at the cost of reducing the effective data rate. On the other extreme, not using any ECC (i.e., a code-rate equal to 1) uses the full channel for information transfer purposes, at the cost of leaving the bits without any additional protection.
One interesting question is the following: how efficient in terms of information transfer can an ECC be that has a negligible decoding error rate? This question was answered by Claude Shannon with his second theorem, which says that the channel capacity is the maximum bit rate achievable by any ECC whose error rate tends to zero:[12] His proof relies on Gaussian random coding, which is not suitable to real-world applications. The upper bound given by Shannon’s work inspired a long journey in designing ECCs that can come close to the ultimate performance boundary. Various codes today can attain almost the Shannon limit. However, capacity achieving ECCs are usually extremely complex to implement.
The most popular ECCs have a trade-off between performance and computational complexity. Usually, their parameters give a range of possible code rates, which can be optimized depending on the scenario. Usually, this optimization is done in order to achieve a low decoding error probability while minimizing the impact to the data rate. Another criterion for optimizing the code rate is to balance low error rate and retransmissions number in order to the energy cost of the communication.[13]
Concatenated ECC codes for improved performance[edit]
Classical (algebraic) block codes and convolutional codes are frequently combined in concatenated coding schemes in which a short constraint-length Viterbi-decoded convolutional code does most of the work and a block code (usually Reed–Solomon) with larger symbol size and block length «mops up» any errors made by the convolutional decoder. Single pass decoding with this family of error correction codes can yield very low error rates, but for long range transmission conditions (like deep space) iterative decoding is recommended.
Concatenated codes have been standard practice in satellite and deep space communications since Voyager 2 first used the technique in its 1986 encounter with Uranus. The Galileo craft used iterative concatenated codes to compensate for the very high error rate conditions caused by having a failed antenna.
Low-density parity-check (LDPC)[edit]
Low-density parity-check (LDPC) codes are a class of highly efficient linear block
codes made from many single parity check (SPC) codes. They can provide performance very close to the channel capacity (the theoretical maximum) using an iterated soft-decision decoding approach, at linear time complexity in terms of their block length. Practical implementations rely heavily on decoding the constituent SPC codes in parallel.
LDPC codes were first introduced by Robert G. Gallager in his PhD thesis in 1960,
but due to the computational effort in implementing encoder and decoder and the introduction of Reed–Solomon codes,
they were mostly ignored until the 1990s.
LDPC codes are now used in many recent high-speed communication standards, such as DVB-S2 (Digital Video Broadcasting – Satellite – Second Generation), WiMAX (IEEE 802.16e standard for microwave communications), High-Speed Wireless LAN (IEEE 802.11n),[14] 10GBase-T Ethernet (802.3an) and G.hn/G.9960 (ITU-T Standard for networking over power lines, phone lines and coaxial cable). Other LDPC codes are standardized for wireless communication standards within 3GPP MBMS (see fountain codes).
Turbo codes[edit]
Turbo coding is an iterated soft-decoding scheme that combines two or more relatively simple convolutional codes and an interleaver to produce a block code that can perform to within a fraction of a decibel of the Shannon limit. Predating LDPC codes in terms of practical application, they now provide similar performance.
One of the earliest commercial applications of turbo coding was the CDMA2000 1x (TIA IS-2000) digital cellular technology developed by Qualcomm and sold by Verizon Wireless, Sprint, and other carriers. It is also used for the evolution of CDMA2000 1x specifically for Internet access, 1xEV-DO (TIA IS-856). Like 1x, EV-DO was developed by Qualcomm, and is sold by Verizon Wireless, Sprint, and other carriers (Verizon’s marketing name for 1xEV-DO is Broadband Access, Sprint’s consumer and business marketing names for 1xEV-DO are Power Vision and Mobile Broadband, respectively).
Local decoding and testing of codes[edit]
Sometimes it is only necessary to decode single bits of the message, or to check whether a given signal is a codeword, and do so without looking at the entire signal. This can make sense in a streaming setting, where codewords are too large to be classically decoded fast enough and where only a few bits of the message are of interest for now. Also such codes have become an important tool in computational complexity theory, e.g., for the design of probabilistically checkable proofs.
Locally decodable codes are error-correcting codes for which single bits of the message can be probabilistically recovered by only looking at a small (say constant) number of positions of a codeword, even after the codeword has been corrupted at some constant fraction of positions. Locally testable codes are error-correcting codes for which it can be checked probabilistically whether a signal is close to a codeword by only looking at a small number of positions of the signal.
Interleaving[edit]
«Interleaver» redirects here. For the fiber-optic device, see optical interleaver.
A short illustration of interleaving idea
Interleaving is frequently used in digital communication and storage systems to improve the performance of forward error correcting codes. Many communication channels are not memoryless: errors typically occur in bursts rather than independently. If the number of errors within a code word exceeds the error-correcting code’s capability, it fails to recover the original code word. Interleaving alleviates this problem by shuffling source symbols across several code words, thereby creating a more uniform distribution of errors.[15] Therefore, interleaving is widely used for burst error-correction.
The analysis of modern iterated codes, like turbo codes and LDPC codes, typically assumes an independent distribution of errors.[16] Systems using LDPC codes therefore typically employ additional interleaving across the symbols within a code word.[17]
For turbo codes, an interleaver is an integral component and its proper design is crucial for good performance.[15][18] The iterative decoding algorithm works best when there are not short cycles in the factor graph that represents the decoder; the interleaver is chosen to avoid short cycles.
Interleaver designs include:
- rectangular (or uniform) interleavers (similar to the method using skip factors described above)
- convolutional interleavers
- random interleavers (where the interleaver is a known random permutation)
- S-random interleaver (where the interleaver is a known random permutation with the constraint that no input symbols within distance S appear within a distance of S in the output).[19]
- a contention-free quadratic permutation polynomial (QPP).[20] An example of use is in the 3GPP Long Term Evolution mobile telecommunication standard.[21]
In multi-carrier communication systems, interleaving across carriers may be employed to provide frequency diversity, e.g., to mitigate frequency-selective fading or narrowband interference.[22]
Example[edit]
Transmission without interleaving:
Error-free message: aaaabbbbccccddddeeeeffffgggg Transmission with a burst error: aaaabbbbccc____deeeeffffgggg
Here, each group of the same letter represents a 4-bit one-bit error-correcting codeword. The codeword cccc is altered in one bit and can be corrected, but the codeword dddd is altered in three bits, so either it cannot be decoded at all or it might be decoded incorrectly.
With interleaving:
Error-free code words: aaaabbbbccccddddeeeeffffgggg Interleaved: abcdefgabcdefgabcdefgabcdefg Transmission with a burst error: abcdefgabcd____bcdefgabcdefg Received code words after deinterleaving: aa_abbbbccccdddde_eef_ffg_gg
In each of the codewords «aaaa», «eeee», «ffff», and «gggg», only one bit is altered, so one-bit error-correcting code will decode everything correctly.
Transmission without interleaving:
Original transmitted sentence: ThisIsAnExampleOfInterleaving Received sentence with a burst error: ThisIs______pleOfInterleaving
The term «AnExample» ends up mostly unintelligible and difficult to correct.
With interleaving:
Transmitted sentence: ThisIsAnExampleOfInterleaving... Error-free transmission: TIEpfeaghsxlIrv.iAaenli.snmOten. Received sentence with a burst error: TIEpfe______Irv.iAaenli.snmOten. Received sentence after deinterleaving: T_isI_AnE_amp_eOfInterle_vin_...
No word is completely lost and the missing letters can be recovered with minimal guesswork.
Disadvantages of interleaving[edit]
Use of interleaving techniques increases total delay. This is because the entire interleaved block must be received before the packets can be decoded.[23] Also interleavers hide the structure of errors; without an interleaver, more advanced decoding algorithms can take advantage of the error structure and achieve more reliable communication than a simpler decoder combined with an interleaver[citation needed]. An example of such an algorithm is based on neural network[24] structures.
Software for error-correcting codes[edit]
Simulating the behaviour of error-correcting codes (ECCs) in software is a common practice to design, validate and improve ECCs. The upcoming wireless 5G standard raises a new range of applications for the software ECCs: the Cloud Radio Access Networks (C-RAN) in a Software-defined radio (SDR) context. The idea is to directly use software ECCs in the communications. For instance in the 5G, the software ECCs could be located in the cloud and the antennas connected to this computing resources: improving this way the flexibility of the communication network and eventually increasing the energy efficiency of the system.
In this context, there are various available Open-source software listed below (non exhaustive).
- AFF3CT(A Fast Forward Error Correction Toolbox): a full communication chain in C++ (many supported codes like Turbo, LDPC, Polar codes, etc.), very fast and specialized on channel coding (can be used as a program for simulations or as a library for the SDR).
- IT++: a C++ library of classes and functions for linear algebra, numerical optimization, signal processing, communications, and statistics.
- OpenAir: implementation (in C) of the 3GPP specifications concerning the Evolved Packet Core Networks.
List of error-correcting codes[edit]
| Distance | Code |
|---|---|
| 2 (single-error detecting) | Parity |
| 3 (single-error correcting) | Triple modular redundancy |
| 3 (single-error correcting) | perfect Hamming such as Hamming(7,4) |
| 4 (SECDED) | Extended Hamming |
| 5 (double-error correcting) | |
| 6 (double-error correct-/triple error detect) | Nordstrom-Robinson code |
| 7 (three-error correcting) | perfect binary Golay code |
| 8 (TECFED) | extended binary Golay code |
- AN codes
- BCH code, which can be designed to correct any arbitrary number of errors per code block.
- Barker code used for radar, telemetry, ultra sound, Wifi, DSSS mobile phone networks, GPS etc.
- Berger code
- Constant-weight code
- Convolutional code
- Expander codes
- Group codes
- Golay codes, of which the Binary Golay code is of practical interest
- Goppa code, used in the McEliece cryptosystem
- Hadamard code
- Hagelbarger code
- Hamming code
- Latin square based code for non-white noise (prevalent for example in broadband over powerlines)
- Lexicographic code
- Linear Network Coding, a type of erasure correcting code across networks instead of point-to-point links
- Long code
- Low-density parity-check code, also known as Gallager code, as the archetype for sparse graph codes
- LT code, which is a near-optimal rateless erasure correcting code (Fountain code)
- m of n codes
- Nordstrom-Robinson code, used in Geometry and Group Theory[25]
- Online code, a near-optimal rateless erasure correcting code
- Polar code (coding theory)
- Raptor code, a near-optimal rateless erasure correcting code
- Reed–Solomon error correction
- Reed–Muller code
- Repeat-accumulate code
- Repetition codes, such as Triple modular redundancy
- Spinal code, a rateless, nonlinear code based on pseudo-random hash functions[26]
- Tornado code, a near-optimal erasure correcting code, and the precursor to Fountain codes
- Turbo code
- Walsh–Hadamard code
- Cyclic redundancy checks (CRCs) can correct 1-bit errors for messages at most
bits long for optimal generator polynomials of degree
, see Mathematics of cyclic redundancy checks#Bitfilters
See also[edit]
- Code rate
- Erasure codes
- Soft-decision decoder
- Burst error-correcting code
- Error detection and correction
- Error-correcting codes with feedback
References[edit]
- ^ Charles Wang; Dean Sklar; Diana Johnson (Winter 2001–2002). «Forward Error-Correction Coding». Crosslink. The Aerospace Corporation. 3 (1). Archived from the original on 14 March 2012. Retrieved 5 March 2006.
- ^ Charles Wang; Dean Sklar; Diana Johnson (Winter 2001–2002). «Forward Error-Correction Coding». Crosslink. The Aerospace Corporation. 3 (1). Archived from the original on 14 March 2012. Retrieved 5 March 2006.
How Forward Error-Correcting Codes Work]
- ^ a b Maunder, Robert (2016). «Overview of Channel Coding».
- ^ Glover, Neal; Dudley, Trent (1990). Practical Error Correction Design For Engineers (Revision 1.1, 2nd ed.). CO, USA: Cirrus Logic. ISBN 0-927239-00-0.
- ^ a b Hamming, Richard Wesley (April 1950). «Error Detecting and Error Correcting Codes». Bell System Technical Journal. USA: AT&T. 29 (2): 147–160. doi:10.1002/j.1538-7305.1950.tb00463.x. S2CID 61141773.
- ^ «Hamming codes for NAND flash memory devices» Archived 21 August 2016 at the Wayback Machine. EE Times-Asia. Apparently based on «Micron Technical Note TN-29-08: Hamming Codes for NAND Flash Memory Devices». 2005. Both say: «The Hamming algorithm is an industry-accepted method for error detection and correction in many SLC NAND flash-based applications.»
- ^ a b «What Types of ECC Should Be Used on Flash Memory?» (Application note). Spansion. 2011.
Both Reed–Solomon algorithm and BCH algorithm are common ECC choices for MLC NAND flash. … Hamming based block codes are the most commonly used ECC for SLC…. both Reed–Solomon and BCH are able to handle multiple errors and are widely used on MLC flash.
- ^ Jim Cooke (August 2007). «The Inconvenient Truths of NAND Flash Memory» (PDF). p. 28.
For SLC, a code with a correction threshold of 1 is sufficient. t=4 required … for MLC.
- ^ Baldi, M.; Chiaraluce, F. (2008). «A Simple Scheme for Belief Propagation Decoding of BCH and RS Codes in Multimedia Transmissions». International Journal of Digital Multimedia Broadcasting. 2008: 1–12. doi:10.1155/2008/957846.
- ^ Shah, Gaurav; Molina, Andres; Blaze, Matt (2006). «Keyboards and covert channels». USENIX. Retrieved 20 December 2018.
- ^ Tse, David; Viswanath, Pramod (2005), Fundamentals of Wireless Communication, Cambridge University Press, UK
- ^ Shannon, C. E. (1948). «A mathematical theory of communication» (PDF). Bell System Technical Journal. 27 (3–4): 379–423 & 623–656. doi:10.1002/j.1538-7305.1948.tb01338.x. hdl:11858/00-001M-0000-002C-4314-2.
- ^ Rosas, F.; Brante, G.; Souza, R. D.; Oberli, C. (2014). «Optimizing the code rate for achieving energy-efficient wireless communications». Proceedings of the IEEE Wireless Communications and Networking Conference (WCNC). pp. 775–780. doi:10.1109/WCNC.2014.6952166. ISBN 978-1-4799-3083-8.
- ^ IEEE Standard, section 20.3.11.6 «802.11n-2009» Archived 3 February 2013 at the Wayback Machine, IEEE, 29 October 2009, accessed 21 March 2011.
- ^ a b Vucetic, B.; Yuan, J. (2000). Turbo codes: principles and applications. Springer Verlag. ISBN 978-0-7923-7868-6.
- ^ Luby, Michael; Mitzenmacher, M.; Shokrollahi, A.; Spielman, D.; Stemann, V. (1997). «Practical Loss-Resilient Codes». Proc. 29th Annual Association for Computing Machinery (ACM) Symposium on Theory of Computation.
- ^ «Digital Video Broadcast (DVB); Second generation framing structure, channel coding and modulation systems for Broadcasting, Interactive Services, News Gathering and other satellite broadband applications (DVB-S2)». En 302 307. ETSI (V1.2.1). April 2009.
- ^ Andrews, K. S.; Divsalar, D.; Dolinar, S.; Hamkins, J.; Jones, C. R.; Pollara, F. (November 2007). «The Development of Turbo and LDPC Codes for Deep-Space Applications». Proceedings of the IEEE. 95 (11): 2142–2156. doi:10.1109/JPROC.2007.905132. S2CID 9289140.
- ^ Dolinar, S.; Divsalar, D. (15 August 1995). «Weight Distributions for Turbo Codes Using Random and Nonrandom Permutations». TDA Progress Report. 122: 42–122. Bibcode:1995TDAPR.122…56D. CiteSeerX 10.1.1.105.6640.
- ^ Takeshita, Oscar (2006). «Permutation Polynomial Interleavers: An Algebraic-Geometric Perspective». IEEE Transactions on Information Theory. 53 (6): 2116–2132. arXiv:cs/0601048. Bibcode:2006cs……..1048T. doi:10.1109/TIT.2007.896870. S2CID 660.
- ^ 3GPP TS 36.212, version 8.8.0, page 14
- ^ «Digital Video Broadcast (DVB); Frame structure, channel coding and modulation for a second generation digital terrestrial television broadcasting system (DVB-T2)». En 302 755. ETSI (V1.1.1). September 2009.
- ^ Techie (3 June 2010). «Explaining Interleaving». W3 Techie Blog. Retrieved 3 June 2010.
- ^ Krastanov, Stefan; Jiang, Liang (8 September 2017). «Deep Neural Network Probabilistic Decoder for Stabilizer Codes». Scientific Reports. 7 (1): 11003. arXiv:1705.09334. Bibcode:2017NatSR…711003K. doi:10.1038/s41598-017-11266-1. PMC 5591216. PMID 28887480.
- ^ Nordstrom, A.W.; Robinson, J.P. (1967), «An optimum nonlinear code», Information and Control, 11 (5–6): 613–616, doi:10.1016/S0019-9958(67)90835-2
- ^ Perry, Jonathan; Balakrishnan, Hari; Shah, Devavrat (2011). «Rateless Spinal Codes». Proceedings of the 10th ACM Workshop on Hot Topics in Networks. pp. 1–6. doi:10.1145/2070562.2070568. hdl:1721.1/79676. ISBN 9781450310598.
Further reading[edit]
- MacWilliams, Florence Jessiem; Sloane, Neil James Alexander (2007) [1977]. Written at AT&T Shannon Labs, Florham Park, New Jersey, USA. The Theory of Error-Correcting Codes. North-Holland Mathematical Library. Vol. 16 (digital print of 12th impression, 1st ed.). Amsterdam / London / New York / Tokyo: North-Holland / Elsevier BV. ISBN 978-0-444-85193-2. LCCN 76-41296. (xxii+762+6 pages)
- Clark, Jr., George C.; Cain, J. Bibb (1981). Error-Correction Coding for Digital Communications. New York, USA: Plenum Press. ISBN 0-306-40615-2.
- Arazi, Benjamin (1987). Swetman, Herb (ed.). A Commonsense Approach to the Theory of Error Correcting Codes. MIT Press Series in Computer Systems. Vol. 10 (1 ed.). Cambridge, Massachusetts, USA / London, UK: Massachusetts Institute of Technology. ISBN 0-262-01098-4. LCCN 87-21889. (x+2+208+4 pages)
- Wicker, Stephen B. (1995). Error Control Systems for Digital Communication and Storage. Englewood Cliffs, New Jersey, USA: Prentice-Hall. ISBN 0-13-200809-2.
- Wilson, Stephen G. (1996). Digital Modulation and Coding. Englewood Cliffs, New Jersey, USA: Prentice-Hall. ISBN 0-13-210071-1.
- «Error Correction Code in Single Level Cell NAND Flash memories» 2007-02-16
- «Error Correction Code in NAND Flash memories» 2004-11-29
- Observations on Errors, Corrections, & Trust of Dependent Systems, by James Hamilton, 2012-02-26
- Sphere Packings, Lattices and Groups, By J. H. Conway, Neil James Alexander Sloane, Springer Science & Business Media, 2013-03-09 – Mathematics – 682 pages.
External links[edit]
- Morelos-Zaragoza, Robert (2004). «The Correcting Codes (ECC) Page». Retrieved 5 March 2006.
- lpdec: library for LP decoding and related things (Python)
схема управления ошибками в данных по зашумленным каналам связи
В вычислениях, телекоммуникации, теория информации и теория кодирования, код исправления ошибок, иногда код исправления ошибок, (ECC ) используется для контроля ошибок в данных по ненадежным или зашумленным каналам связи. Основная идея заключается в том, что отправитель кодирует сообщение с помощью избыточной информации в форме ECC. Избыточность позволяет получателю обнаруживать ограниченное количество ошибок, которые могут возникать в любом месте сообщения, и часто исправлять эти ошибки без повторной передачи. Американский математик Ричард Хэмминг был пионером в этой области в 1940-х годах и изобрел первый исправляющий ошибки код в 1950 году: код Хэмминга (7,4).
ECC контрастирует с обнаружением ошибок. в том, что обнаруженные ошибки можно исправить, а не просто обнаружить. Преимущество состоит в том, что системе, использующей ECC, не требуется обратный канал для запроса повторной передачи данных при возникновении ошибки. Обратной стороной является то, что к сообщению добавляются фиксированные накладные расходы, что требует более высокой полосы пропускания прямого канала. Таким образом, ECC применяется в ситуациях, когда повторные передачи являются дорогостоящими или невозможными, например, при односторонних каналах связи и при передаче на несколько приемников в многоадресной передаче. Соединения с длительной задержкой также выигрывают; в случае спутника, вращающегося вокруг Урана, повторная передача из-за ошибок может вызвать задержку в пять часов. Информация ECC обычно добавляется к запоминающим устройствам для восстановления поврежденных данных, широко используется в модемах и используется в системах, где основной памятью является память ECC.
Обработка ЕСС в приемнике может применяться к цифровому потоку битов или к демодуляции несущей с цифровой модуляцией. В последнем случае ECC является неотъемлемой частью начального аналого-цифрового преобразования в приемнике. Декодер Витерби реализует алгоритм мягкого решения для демодуляции цифровых данных из аналогового сигнала, искаженного шумом. Многие кодеры / декодеры ECC также могут генерировать сигнал с коэффициентом ошибок по битам (BER), который можно использовать в качестве обратной связи для точной настройки аналоговой приемной электроники.
Максимальная доля ошибок или отсутствующих битов, которые могут быть исправлены, определяется конструкцией кода ECC, поэтому разные коды исправления ошибок подходят для разных условий. Как правило, более сильный код вызывает большую избыточность, которую необходимо передавать с использованием доступной полосы пропускания, что снижает эффективную скорость передачи данных при одновременном улучшении принимаемого эффективного отношения сигнал / шум. Теорема кодирования с шумом канала из Клод Шеннон отвечает на вопрос о том, какая полоса пропускания остается для передачи данных при использовании наиболее эффективного кода, который сводит вероятность ошибки декодирования к нулю. Это устанавливает границы теоретической максимальной скорости передачи информации канала с некоторым заданным базовым уровнем шума. Однако это доказательство неконструктивно и, следовательно, не дает представления о том, как создать код, обеспечивающий производительность. После многих лет исследований некоторые современные системы ECC сегодня очень близки к теоретическому максимуму.
Содержание
- 1 Прямое исправление ошибок
- 2 Как это работает
- 3 Усреднение шума для уменьшения количества ошибок
- 4 Типы ECC
- 5 Кодовая скорость и компромисс между надежностью и скоростью передачи данных
- 6 Составные коды ECC для повышения производительности
- 7 Проверка четности с низкой плотностью (LDPC)
- 8 Турбо-коды
- 9 Локальное декодирование и тестирование кодов
- 10 Чередование
- 10.1 Пример
- 10.2 Недостатки чередования
- 11 Программное обеспечение для кодов исправления ошибок
- 12 Список кодов исправления ошибок
- 13 См. Также
- 14 Ссылки
- 15 Дополнительная литература
- 16 Внешние ссылки
Прямое исправление ошибок
В электросвязи, теории информации и теории кодирования, прямое исправление ошибок (FEC ) или канальное кодирование — это метод, используемый для контроля ошибок в передаче данных по ненадежным или зашумленным каналам связи. Основная идея заключается в том, что отправитель кодирует сообщение с помощью избыточного способа, чаще всего с помощью ECC.
Избыточность позволяет получателю обнаруживать ограниченное количество ошибок, которые могут возникнуть в любом месте сообщения, и часто исправлять эти ошибки без повторной передачи. FEC дает приемнику возможность исправлять ошибки без необходимости использования обратного канала для запроса повторной передачи данных, но за счет фиксированной более высокой полосы пропускания прямого канала. Поэтому FEC применяется в ситуациях, когда повторные передачи являются дорогостоящими или невозможными, например, при односторонних каналах связи и при передаче на несколько приемников в многоадресной передаче. Информация FEC обычно добавляется к запоминающим устройствам (магнитным, оптическим и твердотельным / флэш-накопителям) для восстановления поврежденных данных, широко используется в модемах, используется в системах, где первичной памятью является память ECC, и в ситуациях широковещательной передачи, когда приемник не имеет возможности запрашивать повторную передачу или это может вызвать значительную задержку. Например, в случае спутника, вращающегося вокруг Урана, повторная передача из-за ошибок декодирования может вызвать задержку не менее 5 часов.
Обработка FEC в приемнике может применяться к цифровому битовому потоку или при демодуляции несущей с цифровой модуляцией. Для последнего FEC является неотъемлемой частью начального аналого-цифрового преобразования в приемнике. Декодер Витерби реализует алгоритм мягкого решения для демодуляции цифровых данных из аналогового сигнала, искаженного шумом. Многие кодеры FEC могут также генерировать сигнал с коэффициентом ошибок по битам (BER), который можно использовать в качестве обратной связи для точной настройки аналоговой приемной электроники.
Максимальная доля ошибок или недостающих битов, которые могут быть исправлены, определяется конструкцией ECC, поэтому разные коды прямого исправления ошибок подходят для разных условий. Как правило, более сильный код вызывает большую избыточность, которую необходимо передавать с использованием доступной полосы пропускания, что снижает эффективную скорость передачи данных при одновременном улучшении принимаемого эффективного отношения сигнал / шум. Теорема кодирования канала с шумом Клода Шеннона отвечает на вопрос о том, какая полоса пропускания остается для передачи данных при использовании наиболее эффективного кода, который обращает вероятность ошибки декодирования в ноль. Это устанавливает границы теоретической максимальной скорости передачи информации канала с некоторым заданным базовым уровнем шума. Его доказательство неконструктивно и, следовательно, не дает понимания того, как создать код, обеспечивающий производительность. Однако после многих лет исследований некоторые передовые системы FEC, такие как полярный код, достигают пропускной способности канала Шеннона при гипотезе кадра бесконечной длины.
Как это работает
ECC достигается путем добавления избыточности к передаваемой информации с использованием алгоритма. Избыточный бит может быть сложной функцией многих исходных информационных битов. Исходная информация может появляться или не появляться буквально в закодированном выводе; коды, которые включают немодифицированный ввод в вывод, являются систематическими, тогда как те, которые не включают, являются несистематическими .
Упрощенный пример ECC — передача каждого бита данных 3 раза, что известно как код повторения (3,1) . Через шумный канал приемник может видеть 8 вариантов вывода, см. Таблицу ниже.
| Получен триплет | Интерпретируется как |
|---|---|
| 000 | 0 (без ошибок) |
| 001 | 0 |
| 010 | 0 |
| 100 | 0 |
| 111 | 1 (без ошибок) |
| 110 | 1 |
| 101 | 1 |
| 011 | 1 |
Это позволяет исправить ошибку в любой из трех выборок «большинством голосов» или «демократическим голосованием». Корректирующая способность этого ECC:
- До 1 бита триплета с ошибкой или
- до 2 битов триплета пропущены (случаи не показаны в таблице).
Хотя прост в реализации и Это широко используемое тройное модульное резервирование является относительно неэффективным ECC. Более совершенные коды ECC обычно проверяют несколько последних десятков или даже несколько последних сотен ранее принятых битов, чтобы определить, как декодировать текущую небольшую группу битов (обычно в группах от 2 до 8 бит).
Усреднение шума для уменьшения ошибок
Можно сказать, что ECC работает посредством «усреднения шума»; поскольку каждый бит данных влияет на многие передаваемые символы, искажение одних символов шумом обычно позволяет извлекать исходные пользовательские данные из других неповрежденных принятых символов, которые также зависят от тех же пользовательских данных.
- Из-за этого эффекта «объединения рисков» цифровые системы связи, использующие ECC, как правило, работают значительно выше определенного минимального отношения сигнал / шум, а не ниже него.
- Эта тенденция «все или ничего» — эффект обрыва — становится более выраженной по мере использования более сильных кодов, которые более близко подходят к теоретическому пределу Шеннона.
- Чередование данных, закодированных с помощью ECC, может уменьшить все или ничего свойства переданных кодов ECC, когда ошибки канала имеют тенденцию возникать в пакетах. Однако у этого метода есть ограничения; его лучше всего использовать для узкополосных данных.
Большинство телекоммуникационных систем используют фиксированный канальный код, рассчитанный на ожидаемый наихудший случай частоты ошибок по битам, а затем вообще не работают если частота ошибок по битам станет еще хуже. Однако некоторые системы адаптируются к данным условиям ошибки канала: некоторые экземпляры гибридного автоматического запроса на повторение используют фиксированный метод ECC, пока ECC может обрабатывать частоту ошибок, затем переключаются на ARQ когда частота ошибок становится слишком высокой; адаптивная модуляция и кодирование использует различные скорости ECC, добавляя больше битов исправления ошибок на пакет, когда в канале более высокие частоты ошибок, или удаляя их, когда они не нужны.
Типы ECC

Двумя основными категориями кодов ECC являются блочные коды и сверточные коды.
- Блочные коды работают с блоками фиксированного размера (пакетами) битов или символов заранее определенного размера. Практические блочные коды обычно могут быть жестко декодированы за полиномиальное время до их длины блока.
- Сверточные коды работают с битовыми или символьными потоками произвольной длины. Чаще всего они программно декодируются с помощью алгоритма Витерби, хотя иногда используются и другие алгоритмы. Декодирование Витерби обеспечивает асимптотически оптимальную эффективность декодирования с увеличением длины ограничения сверточного кода, но за счет экспоненциально возрастающей сложности. Завершенный сверточный код также является «блочным кодом» в том смысле, что он кодирует блок входных данных, но размер блока сверточного кода, как правило, произвольный, в то время как блочные коды имеют фиксированный размер, определяемый их алгебраическими характеристиками. Типы завершения для сверточных кодов включают в себя «бит в конце» и «сброс битов».
Существует много типов блочных кодов; Кодирование Рида-Соломона примечательно тем, что оно широко используется в компакт-дисках, DVD и жестких дисках. Другие примеры классических блочных кодов включают Голея, BCH, многомерную четность и коды Хэмминга.
ECC Хэмминга обычно используются для исправления NAND flash ошибки памяти. Это обеспечивает исправление однобитовых ошибок и обнаружение двухбитовых ошибок. Коды Хэмминга подходят только для более надежной одноуровневой ячейки (SLC) NAND. Более плотная многоуровневая ячейка (MLC) NAND может использовать многобитовый корректирующий ECC, такой как BCH или Reed-Solomon. NOR Flash обычно не использует никакого исправления ошибок.
Классические блочные коды обычно декодируются с использованием алгоритмов жесткого решения, что означает, что для каждого входного и выходного сигнала принимается жесткое решение, будет ли он соответствует единице или нулю бит. Напротив, сверточные коды обычно декодируются с использованием алгоритмов мягкого решения, таких как алгоритмы Витерби, MAP или BCJR, которые обрабатывают (дискретизированные) аналоговые сигналы и которые допускают гораздо более высокие ошибки — производительность коррекции, чем декодирование с жестким решением.
Почти все классические блочные коды применяют алгебраические свойства конечных полей. Поэтому классические блочные коды часто называют алгебраическими кодами.
В отличие от классических блочных кодов, которые часто определяют способность обнаружения или исправления ошибок, многие современные блочные коды, такие как коды LDPC, не имеют таких гарантий. Вместо этого современные коды оцениваются с точки зрения их частоты ошибок по битам.
Большинство кодов прямого исправления ошибок исправляют только перевороты битов, но не вставки или удаления битов. В этой настройке расстояние Хэмминга является подходящим способом измерения коэффициента битовых ошибок. Несколько кодов прямого исправления ошибок предназначены для исправления вставки и удаления битов, например, коды маркеров и коды водяных знаков. Расстояние Левенштейна является более подходящим способом измерения частоты ошибок по битам при использовании таких кодов.
Кодовая скорость и компромисс между надежностью и скоростью передачи данных
Фундаментальный принцип ECC состоит в добавлении избыточных битов, чтобы помочь декодеру узнать истинное сообщение, которое было закодировано передатчик. Кодовая скорость данной системы ЕСС определяется как соотношение между количеством информационных битов и общим количеством битов (то есть информацией плюс биты избыточности) в данном коммуникационном пакете. Кодовая скорость, следовательно, является действительным числом. Низкая кодовая скорость, близкая к нулю, подразумевает сильный код, который использует много избыточных битов для достижения хорошей производительности, в то время как большая кодовая скорость, близкая к 1, подразумевает слабый код.
Избыточные биты, защищающие информацию, должны передаваться с использованием тех же коммуникационных ресурсов, которые они пытаются защитить. Это вызывает фундаментальный компромисс между надежностью и скоростью передачи данных. В одном крайнем случае сильный код (с низкой кодовой скоростью) может вызвать значительное увеличение SNR приемника (отношение сигнал / шум), уменьшая частоту ошибок по битам, за счет снижения эффективной скорости передачи данных. С другой стороны, без использования какого-либо ECC (то есть кодовой скорости, равной 1) используется полный канал для целей передачи информации за счет того, что биты остаются без какой-либо дополнительной защиты.
Один интересный вопрос заключается в следующем: насколько эффективным с точки зрения передачи информации может быть ECC, имеющий незначительную частоту ошибок декодирования? На этот вопрос ответил Клод Шеннон с его второй теоремой, которая гласит, что пропускная способность канала — это максимальная скорость передачи данных, достижимая для любого ECC, частота ошибок которого стремится к нулю: его доказательство основано на гауссовском случайном кодировании, которое не подходит для реального мира. Приложения. Верхняя граница, заданная работой Шеннона, вдохновила на долгий путь к разработке ECC, которые могут приблизиться к пределу конечных характеристик. Различные коды сегодня могут достигать почти предела Шеннона. Однако ECC, обеспечивающие пропускную способность, обычно чрезвычайно сложно реализовать.
Наиболее популярные ECC имеют компромисс между производительностью и вычислительной сложностью. Обычно их параметры дают диапазон возможных кодовых скоростей, которые можно оптимизировать в зависимости от сценария. Обычно эта оптимизация выполняется для достижения низкой вероятности ошибки декодирования при минимальном влиянии на скорость передачи данных. Другим критерием оптимизации кодовой скорости является уравновешивание низкой частоты ошибок и количества повторных передач с учетом энергетических затрат на связь.
Составные коды ECC для повышения производительности
Классические (алгебраические) блочные коды а сверточные коды часто комбинируются в схемах конкатенированного кодирования, в которых сверточный код, декодированный по Витерби с короткой ограниченной длиной, выполняет большую часть работы, а блочный код (обычно Рида-Соломона) с большим размером символа и длиной блока «стирает» любые ошибки, сделанные сверточным декодером. Однопроходное декодирование с использованием этого семейства кодов с исправлением ошибок может дать очень низкий уровень ошибок, но для условий передачи на большие расстояния (например, в глубоком космосе) рекомендуется итеративное декодирование.
Составные коды были стандартной практикой в спутниковой связи и связи в дальнем космосе с тех пор, как «Вояджер-2 » впервые применил эту технику во время встречи с Ураном в 1986 году. Аппарат Galileo использовал итеративные конкатенированные коды для компенсации условий очень высокой частоты ошибок, вызванных отказом антенны.
Проверка на четность с низкой плотностью (LDPC)
Коды с проверкой на четность с низкой плотностью (LDPC) — это класс высокоэффективных линейных блочных кодов, созданных из множества кодов одиночной проверки на четность (SPC). Они могут обеспечить производительность, очень близкую к пропускной способности канала (теоретический максимум), используя подход итеративного декодирования с мягким решением, при линейной временной сложности с точки зрения длины их блока. Практические реализации в значительной степени полагаются на параллельное декодирование составляющих кодов SPC.
Коды LDPC были впервые введены Робертом Г. Галлагером в его докторской диссертации в 1960 году, но из-за вычислительных усилий при реализации кодера и декодера и введения Рида-Соломона коды, они в основном игнорировались до 1990-х годов.
Коды LDPC теперь используются во многих недавних стандартах высокоскоростной связи, таких как DVB-S2 (цифровое видеовещание — спутниковое — второе поколение), WiMAX ( стандарт IEEE 802.16e для микроволновой связи), высокоскоростная беспроводная локальная сеть (IEEE 802.11n ), 10GBase-T Ethernet (802.3an) и G.hn/G.9960 (Стандарт ITU-T для организации сетей по линиям электропередач, телефонным линиям и коаксиальному кабелю). Другие коды LDPC стандартизированы для стандартов беспроводной связи в пределах 3GPP MBMS (см. исходные коды ).
Турбокоды
Турбокодирование — это схема повторяющегося мягкого декодирования, которая объединяет два или более относительно простых сверточных кода и перемежитель для создания блочного кода, который может работать с точностью до долей децибела. предела Шеннона. Предшествующие LDPC-коды с точки зрения практического применения, теперь они обеспечивают аналогичную производительность.
Одним из первых коммерческих приложений турбо-кодирования была технология цифровой сотовой связи CDMA2000 1x (TIA IS-2000), разработанная Qualcomm и продаваемая Verizon Беспроводная связь, Sprint и другие операторы связи. Он также используется для развития CDMA2000 1x специально для доступа в Интернет, 1xEV-DO (TIA IS-856). Как и 1x, EV-DO был разработан Qualcomm и продается Verizon Wireless, Sprint и другими операторами (маркетинговое название Verizon для 1xEV-DO — Широкополосный доступ, потребительские и бизнес-маркетинговые названия компании Sprint для 1xEV-DO — Power Vision и Mobile Broadband соответственно).
Локальное декодирование и тестирование кодов
Иногда необходимо декодировать только отдельные биты сообщения или проверить, является ли данный сигнал кодовым словом, и делать это, не глядя на все сигнал. Это может иметь смысл в настройке потоковой передачи, где кодовые слова слишком велики для того, чтобы их можно было классически декодировать достаточно быстро, и где на данный момент интересны только несколько битов сообщения. Также такие коды стали важным инструментом в теории сложности вычислений, например, для разработки вероятностно проверяемых доказательств.
Локально декодируемые коды являются кодами с исправлением ошибок, для которых отдельные биты сообщение может быть восстановлено вероятностно, если посмотреть только на небольшое (скажем, постоянное) количество позиций кодового слова, даже после того, как кодовое слово было искажено на некоторой постоянной доле позиций. Локально тестируемые коды — это коды с исправлением ошибок, для которых можно вероятностно проверить, близок ли сигнал к кодовому слову, посмотрев только на небольшое количество позиций сигнала.
Чередование

Чередование часто используется в системах цифровой связи и хранения для повышения производительности кодов прямого исправления ошибок. Многие каналы связи не лишены памяти: ошибки обычно возникают в пакетах, а не независимо друг от друга. Если количество ошибок в кодовом слове превышает возможности кода исправления ошибок, ему не удается восстановить исходное кодовое слово. Чередование облегчает эту проблему путем перетасовки исходных символов по нескольким кодовым словам, тем самым создавая более равномерное распределение ошибок. Поэтому перемежение широко используется для пакетной коррекции ошибок.
. Анализ современных повторяющихся кодов, таких как турбокоды и коды LDPC, обычно предполагает независимое распределение ошибок.. Поэтому системы, использующие коды LDPC, обычно используют дополнительное перемежение символов в кодовом слове.
Для турбокодов перемежитель является неотъемлемым компонентом, и его правильная конструкция имеет решающее значение для хорошей производительности. Алгоритм итеративного декодирования работает лучше всего, когда нет коротких циклов в графе коэффициентов, который представляет декодер; перемежитель выбран, чтобы избежать коротких циклов.
Конструкции перемежителя включают:
- прямоугольные (или однородные) перемежители (аналогично методу с использованием коэффициентов пропуска, описанному выше)
- сверточные перемежители
- случайные перемежители (где перемежитель — известная случайная перестановка)
- S-случайный перемежитель (где перемежитель — это известная случайная перестановка с ограничением, что никакие входные символы на расстоянии S не появляются на расстоянии S на выходе).
- бесконфликтный квадратичный многочлен с перестановками (QPP). Пример использования — в стандарте мобильной связи 3GPP Long Term Evolution.
В системах связи с несколькими несущими может использоваться перемежение по несущим для обеспечения частотного разнесения., например, для уменьшения частотно-избирательного замирания или узкополосных помех.
Пример
Передача без перемежения :
Сообщение без ошибок: aaaabbbbccccddddeeeeffffgggg Передача с пакетной ошибкой: aaaabbbbccc____deeeeffffgggg
Здесь каждая группа одинаковых букв представляет 4-битное однобитовое кодовое слово с исправлением ошибок. Кодовое слово cccc изменяется в один бит и может быть исправлено, но кодовое слово dddd изменяется в трех битах, поэтому либо оно не может быть декодировано вообще, либо может быть декодировано неправильно.
С чередованием :
Ошибка- свободные кодовые слова: aaaabbbbccccddddeeeeffffgggg Interleaved: abcdefgabcdefgabcdefgabcdefg Передача с ошибкой пакета: abcdefgabcd____bcdefgabcdefg Полученные кодовые слова после деинтерлейвинга: "aa_abbb_gg2ccd_dd>,", ",", ",", ","Передача без чередования :
Исходное переданное предложение: ThisIsAnExampleOfInterleaving Полученное предложение с пакетной ошибкой: ThisIs______pleOfInterleavingТермин «AnExample» оказывается в основном неразборчивым и трудным для исправления.
С чередованием :
Переданное предложение: ThisIsAnExampleOfInterleaving... Безошибочная передача: TIEpfeaghsxlIrv.iAaenli.snmOten. Получено предложение с пакетной ошибкой: TIEpfe ______ Irv.iAaenli.snmOten. Полученное предложение после деинтерлейвинга: T_isI_AnE_amp_eOfInterle_vin _...Ни одно слово не потеряно полностью, а недостающие буквы можно восстановить с минимальными догадками.
Недостатки чередования
Использование методов чередования увеличивает общую задержку. Это связано с тем, что весь чередующийся блок должен быть принят до того, как пакеты могут быть декодированы. Также перемежители скрывают структуру ошибок; Без перемежителя более совершенные алгоритмы декодирования могут использовать структуру ошибок и обеспечивать более надежную связь, чем более простой декодер, объединенный с перемежителем. Пример такого алгоритма основан на структурах нейронной сети .
Программное обеспечение для кодов с исправлением ошибок
Моделирование поведения кодов с исправлением ошибок (ECC) в программном обеспечении является обычной практикой для разработки, проверки и улучшения кодов ECC. Предстоящий стандарт беспроводной связи 5G поднимает новый диапазон приложений для программных ECC: Облачные сети радиодоступа (C-RAN) в контексте Программно-определяемого радио (SDR). Идея состоит в том, чтобы напрямую использовать программные ECC в коммуникациях. Например, в 5G программные ECC могут быть расположены в облаке, а антенны могут быть подключены к этим вычислительным ресурсам: таким образом повышается гибкость сети связи и, в конечном итоге, повышается энергоэффективность системы.
В этом контексте существует различное доступное программное обеспечение с открытым исходным кодом, перечисленное ниже (не является исчерпывающим).
- AFF3CT (Панель инструментов быстрого исправления ошибок): полная цепочка связи на C ++ (многие поддерживаемые коды, такие как Turbo, LDPC, полярные коды и т. Д.), Очень быстрая и специализированная на канальном кодировании (может использоваться как программа для моделирования или как библиотека для SDR).
- IT ++ : библиотека классов и функций C ++ для линейной алгебры, числовой оптимизации, обработки сигналов, связи и статистики.
- OpenAir : реализация (на языке C) спецификаций 3GPP, касающихся Evolved Packet Core Networks.
Список кодов исправления ошибок
| Расстояние | Код |
|---|---|
| 2 (обнаружение единичной ошибки) | Четность |
| 3 (исправление одиночной ошибки) | Тройное модульное резервирование |
| 3 (исправление одиночной ошибки) | совершенное Хэмминга, такое как Хэмминга (7,4) |
| 4 (SECDED ) | Расширенный Хэмминга |
| 5 (исправление двойной ошибки) | |
| 6 (исправление двойной ошибки / обнаружение тройной ошибки) | |
| 7 (исправление трех ошибок) | совершенный двоичный код Голея |
| 8 (TECFED) | расширенный двоичный код Голея |
- коды AN
- код BCH, который может быть разработан для исправления любого произвольного количества ошибок в кодовом блоке.
- код Бергера
- код постоянного веса
- сверточный код
- Расширительные коды
- Групповые коды
- коды Голея, из которых двоичный код Голея представляет практический интерес
- код Гоппа, используемый в Криптосистема Мак-Элиса
- Код Адамара
- Код Хагельбаргера
- Код Хэмминга
- Код на основе латинского квадрата для небелого шума (преобладающий, например, в широкополосной связи по сравнению с линиями электропередач)
- Лексикографический код
- Линейное сетевое кодирование, тип кода с исправлением стирания в сетях вместо двухточечных ссылок
- Длинный код
- Код проверки четности с низкой плотностью, также известный как код Галлагера, как архетип для кодов разреженного графа
- LT-кода, который является почти оптимальным бесскоростным кодом коррекции стирания (код Фонтана)
- m из n кодов
- Онлайн-код, почти оптимальный код бесскоростной коррекции стирания
- Полярный код (codi ng теория)
- Код Raptor, почти оптимальный код с бесскоростной коррекцией стирания
- Исправление ошибок Рида – Соломона
- Код Рида – Маллера
- Код повторения-накопления
- Коды повторения, например, Тройная модульная избыточность
- Спинальный код, бесскоростной нелинейный код, основанный на псевдослучайных хэш-функциях
- Код Торнадо, почти оптимальный код коррекции стирания, и предшественник кодов Фонтана
- Турбо-код
- код Уолша – Адамара
- Циклические проверки избыточности (CRC) могут исправлять 1-битные ошибки для сообщений не более 2 n - 1 - 1 { displaystyle 2 ^ {n-1} -1}
бит длиной для оптимальных порождающих полиномов степени n { displaystyle n}
, см. Математика циклических проверок избыточности # Битовые фильтры
См. Также
- Скорость кода
- Коды стирания
- Декодер с мягким решением
- Пакетный код исправления ошибок
- Обнаружение и исправление ошибок
- Ошибка -корректирующие коды с обратной связью
Ссылки
Дополнительная литература
- Clark, Jr., George C.; Каин, Дж. Бибб (1981). Кодирование с коррекцией ошибок для цифровой связи. Нью-Йорк, США: Plenum Press. ISBN 0-306-40615-2. ISBN 978-0-306-40615-7.
- Уикер, Стивен Б. (1995). Системы контроля ошибок для цифровой связи и хранения. Энглвуд Клиффс, Нью-Джерси, США: Прентис-Холл. ISBN 0-13-200809-2. ISBN 978-0-13-200809-9.
- Уилсон, Стивен Г. (1996). Цифровая модуляция и кодирование. Энглвуд Клиффс, Нью-Джерси, США: Прентис-Холл. ISBN 0-13-210071-1. ISBN 978-0-13-210071-7.
- "Код коррекции ошибок в одноуровневой ячейке NAND флэш-памяти « 16 февраля 2007 г.
- « Код исправления ошибок во флэш-памяти NAND » 29 ноября 2004 г.
- Наблюдения за ошибками, исправлениями и доверием зависимых систем, Джеймс Гамильтон, 26 февраля 2012 г.
- Сферические упаковки, решетки и группы, Дж. Х. Конвей, NJA Sloane, Springer Science Business Media, 9 марта 2013 г. - Математика - 682 страницы.
Внешние ссылки
- Морелос -Зарагоса, Роберт (2004). "Страница корректирующих кодов (ECC)". Проверено 5 марта 2006 г.
- lpdec: библиотека для декодирования LP и связанных вещей (Python)
ECC, от английского error-correcting code, переводиться на русский язык, как код коррекции ошибок. Встроенная в контроллёры флешек технология, обнаружения и исправления ошибок при передаче данных. ECC способна справиться только с несущественными проблемами, в тяжелых случаях флешка заблокируется на запись данных.
ЗАЧЕМ ЭТО НАДО
Если в эпоху качественных SLC и MLC микросхем флэш-памяти, не было особого смысла обращать внимание на этот механизм исправления ошибок. То сейчас когда в подавляющем количестве флешек, установлена или банально TLC-память или какая-нибудь MLC DownGrade, не стоит пренебрегать настройками ECC-механизма.
Данная технология позволяет продлить жизнь флешки до следующих затыков с ней, ведь не хочется, каждый месяц заново перепрошивать свою флешку.
Еще одной положительной чертой, является вероятность достижения максимального возможного объёма флэш-диска. Он может быть даже выше, чем изначально имел носитель, особенно у флешек с отбракованными микросхемами.
НЕДОСТАТКИ
Чем выше вы установите значение ECC-параметра, тем большую нагрузку он создаст на контроллёр флешки. А это в свою очередь, может негативно сказаться на её производительность, т.е. скорость работы. Также из заметных недостатков, высокой нагрузки, это больший разогрев флешки.
РЕКОМЕНДУЕМЫЕ ЗНАЧЕНИЯ ПАРАМЕТРА ECC
В большинстве утилитах, используется не применяемые в флэш-листах значения (например: 7b/512B и 72b/1K), а суммы определенных параметров. Как правило, в диапазоне равеном от 0 до 15, в некоторых производственных программах, ввиду поддержки крайне некачественной памяти, от 0 до 20. Для посетителей проекта USBDev.ru, я составил следующую таблицу.
| ECC Value | |
|---|---|
| MEMORY TYPE: | ECC: |
| SLC | 1 |
| MLC 32nm, 35nm, 42nm, 50nm, … | 3-4 |
| MLC 24nm, 25nm, 26nm, 32nm | 4-8 |
| MLC 21nm, 20nm, 19nm, … | 8-12 |
| TLC 27nm, 32nm, 43nm, … | 8 |
| TLC 24nm, 21nm, 19nm, … | 12-15 |
В некоторых утилитах, используется другая система координат, к примеру производственный комплекс Dyna для контроллёров SMI. На этот случай, чуть ниже можно обнаружить ссылку на особености настроек у конкретных производителей.
Немного поясню, как следует использовать таблицу данную выше. Так вот, если ваша флешка добротная (хорошо зарекомендовавший себя бренд), то выбирайте минимальное значение из неё. Для подарочных и поддельных флешек, настоятельно советую использовать максимальное значение параметра ECC, для своего типа памяти.
РЕАЛИЗАЦИЯ В ПРОИЗВОДСТВЕННЫХ УТИЛИТАХ
Далеко не во всех утилит, имеется возможность ручной корректировки ECC-опции. Можно сказать что ECC, это такая фитча Sorting-составляющей производственных утилит. Попробую кратко выразить это в таблице, для основных производителей USB-контроллёров.
| ECC Compatible Software | |
|---|---|
| Company: | Tools: |
| ALCOR | AlcorMP_UFD FC MpTool AAMP |
| CHIPSBANK | Chipsbank UMPTool CBM2093 UMPTool CBM2098 UMPTool umptool209X V68 Building Tools |
| INNOSTOR | Innostor MPTool Innostor 917 LFA MP Tool |
| PHISON | UPTool UP19_CTool UP21_CTool UP23_CTool |
| SILICON GO | KingStore Manufacture Tool SiliconGo MPTools SiliconGo MPTool2 |
| SKYMEDI | SK6221 MPTool |
| SMI | Dyna Mass Storage Production Tool |
| СТАТЬИ НА ТЕМУ ECC-КОРРЕКЦИИ | ||
|---|---|---|
| ECC значения для контроллёров Alcor с DownGrade памятью | 2015 | |
| Настройка ECC у контроллёров Silicon Motion (SMI) | 2015 | |
Для ваших вопросов, на проекте USBDev существует форум – FORUM.
This article is based on a talk in Chris Budd’s ongoing Gresham
College lecture series. You can see a video of the talk below and there is another article based on the talk here.
We are surrounded by information and are constantly receiving and transmitting it to other people all over the world. With good reason we can call the 21st century the information age. But whenever a message is being sent, be it over the phone, via the internet, or via satellites that orbit the Earth, there is the danger for errors to creep in. Background noise, technical faults, even cosmic rays can corrupt the message and important information may be lost. Rather amazingly, however, there are ways of encoding a message that allow errors to be detected, and even corrected, automatically. Here is how these codes work.
The 15th century scribe, manuscript illuminator, translator and author Jean Miélot at his desk.
Error detecting codes
The need for the detection of errors has been recognised since the earliest scribes copied manuscripts by hand.
It was important to copy these without error, but to check every word would have been too large a task. Instead various checks were used. For example, when copying the Torah the letters, words, and paragraphs were counted, and then checked against the middle paragraph, word and letter of the original document. If they didn’t match, there was a problem.
Modern digital information is encoded as sequences of 0s and 1s. When transmitting binary information a simple check that is often used involves a so-called hash function, which adds a fixed-length tag to a message. The tag allows the receiver to verify the delivered message by re-computing it and comparing it with the one provided in the message.
A simple example of such a tag comes from including a check or parity digit in each block of data. The simplest example of this method is to add up the digits that make up a message and then append a 1 if the sum is odd and a 0 if it is even. So if the original message is 111 then message sent is 1111, and if the original message is 101, then the message sent is 1010. The effect of this is that every message sent should have digits adding up to an even number. On receiving the transmission the receiving computer will add up the digits, and if the sum is not even then it will record an error.
An example of the use of this technology can be found on the bar codes that are used on nearly all mass-produced consumer goods sold. Such consumer products typically use either UPC-A or EAN-13 codes. UPC-A describes a 12-digit sequence, which is broken into four groups. The first digit of the bar code carries some general information about the item: it can either indicate the nationality of the manufacturer, or describe one of a few other categories, such as the ISBN (book identifier) numbers. The next five digits are a manufacturer’s identification. The five digits that follow are a product identification number, assigned by the manufacturer. The last digit is a check digit, allowing a scanner to validate whether the barcode has been read correctly.
Similar check digits are used in the numbers on your credit card, which are usually a sequence of decimal digits. In this case the Luhn algorithm is used (find out more here).
Error correcting codes
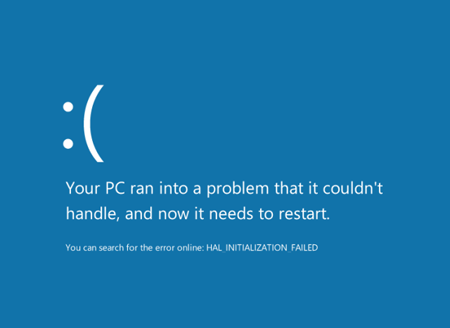
Suppose that we have detected an error in a message we have deceived. How can we proceed to find the correct information contained in the message? There are various approaches to this. One is simply to (effectively) panic: to shut the whole system down and not to proceed until the problem has been fixed. The infamous blue screen of death, pictured below and familiar to many computer operators, is an example of this.
Blue Screen of Death on Windows 8.
The rationale behind this approach is that in some (indeed many) cases it is better to do nothing than to do something which you know is wrong. However, all we can do at this point is to start again from scratch, and we lose all information in the process.
A second approach called the automatic repeat request (ARQ), often used on the Internet, is for the message to be repeated if it is thought to contain an error. We do this all the time. For example if you scan in an item at the supermarket and the scanner does not recognise it, then you simply scan it in again.
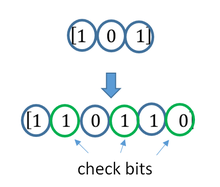
However, this option is not available to us if we are receiving information from, say, a satellite, a mobile phone or from a CD. In this case if we know that an error has been made in transmission then we have to attempt to correct it. The general idea for achieving correction is to add some extra data to a message, which allows us to recover it even after an error has been made. To do this, the transmitter sends the original data, and attaches a fixed number of check bits using an error correcting code (ECC). The idea behind this is to make the symbols from the different characters in the code as different from each other as possible, so that even if one symbol in the code was corrupted by noise it could still be distinguished from other symbols in the code. Error correcting codes were invented in 1947, at Bell Labs, by the American mathematician Richard Hamming.
Illustration of the U.S./European Ocean Surface Topography Mission (OSTM)/Jason-2 satellite in orbit.
Image: NASA-JPL/Caltech
To understand how error correcting codes work we must define the Hamming distance between two binary strings. Suppose that we have two six-bit strings such as 1 1 1 0 1 0 and 1 0 1 1 1 1. Then the Hamming distance is the number of digits which are different. So in this case the Hamming distance is 3. If one bit in a symbol is changed then it has a Hamming distance of one from its original. This change might be due to the action of noise, which has corrupted the message. If two strings are separated by a large Hamming distance, say 3, then they can still be distinguished even if one bit is changed by noise. The idea of the simplest error correcting codes is to exploit this fact by adding extra digits to a binary symbol, so that the symbols are a large Hamming distance apart.
I will illustrate this with a simple example. The numbers from 0 up to 7, written in binary, are:
000 (0) 001 (1) 010 (2) 011 (3)
100 (4) 101 (5) 110 (6) 111 (7)
(You can read more about binary numbers here, or simply believe me.)
Many of these strings are only one Hamming distance apart. For example a small amount of noise could turn the string for 2 into the string for 3. We now add some extra parity digits (we explain the maths behind these later) to these codes to give the code
000 000 (0) 001 110 (1) 010 011 (2) 011 101 (3)
100 101 (4) 101 011 (5) 110 110 (6) 111 000 (7)
The point of doing this is that each of these codes is a Hamming distance of 3 apart. Suppose that the noise is fairly low and has the effect changing one bit of a symbol. Suppose we take the symbol 101 011 for 5 and this changes to (say) 100 011. This is a Hamming distance of one from the original symbol and a distance of at least two from all of the others. So if we receive 100 011 what we do is to find the nearest symbol on our list to this. This must be the original symbol, and we correct 100 011 to 101 011 to read the symbol without error.
Space flight, Facebook and CDs
All error correcting codes use a similar principle to the one above: receive a string, if it’s not on the list
find the closest string on the list to the one received, correct the string to this one. A lot of mathematical sophistication is used to find codes for more symbols which will work in the presence of higher levels of noise. Basically the challenge is to find symbols which are as different from each other as possible. Alongside the development of a code is that of an efficient decoder, which is used to correct corrupted messages fast and reliably.
Another example of an error correcting code is the Reed-Solomon code invented in 1960. The first commercial application in mass-produced consumer products appeared in 1982, with the CD, where two Reed–Solomon codes are used on each track to give even greater redundancy. This is very useful when having to reconstruct the music on a scratched CD.
Today, Reed–Solomon codes are widely implemented in digital storage devices and digital communication standards (for example digital TV), although they are now being replaced by low-density parity-check (LDPC) codes. Reed–Solomon coding is very widely used in mass storage systems to correct the burst errors associated with media defects. This code can correct up to 2 byte errors per 32-byte block. One significant application of Reed–Solomon coding was to encode the digital pictures sent back by the Voyager space probe, which was launched in 1977 and took the first satellite pictures of Jupiter, Saturn and the far planets.
A major recent user of error correction is on Facebook, which is possibly the largest repository of information in the world. It is estimated that 300 million photos are stored on Facebook every day. This information is stored in vast data banks around the world, mostly on spinning discs. Whilst the individual failure rate of a disc is very low, there are so many discs required to store the information that the chance of one of the discs failing at any one time is high. When this happens the data on the disc is recovered efficiently and quickly by using a Reed-Solomon code, so that Facebook can continue without interruption.
Some mathematics
In this last section we will give some mathematical detail for those who are interested. (If you aren’t interested in the details, you can skip to the next article.) It is far from simple to work out codes which are both efficient and robust, and a whole branch of mathematics, coding theory, has been developed to do it.
The Hamming (7,4) code is similar to the Hamming code above, only in this case three parity bits are added to a message with four information bits (rather than a message with three information bits , as above). If 

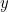
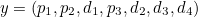
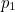
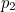
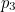
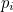
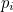
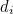
For example, this means that if 
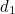
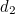
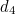



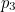
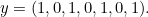
Now suppose that 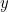
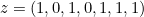
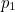

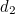
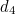
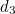
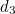
In a more abstract setting
![[ y = G x, ]](https://plus.maths.org/MI/0f3ad8b23b9fbff178e9a0726c0b363d/images/img-0001.png) |
where 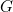

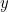
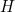
![[ d=Hz ]](https://plus.maths.org/MI/0f3ad8b23b9fbff178e9a0726c0b363d/images/img-0006.png) |
should be the zero vector. If it is not the zero vector, then an error has occurred. The vector 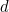
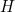
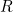
![[ x = R z. ]](https://plus.maths.org/MI/0f3ad8b23b9fbff178e9a0726c0b363d/images/img-0009.png) |
Évariste Galois, drawn from memory by his brother in 1848, sixteen years after his death.
The Reed-Solomon code is more sophisticated in its construction. In the classic implementation of the Reed-Solomon code the original message is mapped to a polynomial with the terms of the message being the coefficients of the polynomial. The transmitted message is then given by evaluating the polynomial at a set of points. As in the Hamming (7,4) code, the Reed-Solomon code is a linear transformation of the original message. Decoding works by finding the best fitting message polynomial. All of the multiplications for the polynomial are performed over mathematical structures called finite fields. The theory behind the construction of these codes uses advanced ideas from the branch of mathematics called Galois theory, which was invented by the French mathematician Évariste Galois
when he was only 19, and at least 150 years before it was used in CD players.
About this article
This article is based on a talk in Budd’s ongoing Gresham
College lecture series. A video of the talk is below and there is another article based on the talk here.
Chris Budd.
Chris Budd OBE is Professor of Applied Mathematics at the University of Bath, Vice President of the Institute of Mathematics and its Applications, Chair of Mathematics for the Royal Institution and an honorary fellow of the British Science Association. He is particularly interested in applying mathematics to the real world and promoting the public understanding of mathematics.
He has co-written the popular mathematics book Mathematics Galore!, published by Oxford University Press, with C. Sangwin, and features in the book 50 Visions of Mathematics ed. Sam Parc.